Advertisements
Advertisements
Question
The length of two sides of a triangle are 7 cm and 9 cm. The length of the third side may lie between ______.
Options
1 cm and 10 cm
2 cm and 8 cm
3 cm and 16 cm
1 cm and 16 cm
Solution
The length of two sides of a triangle are 7 cm and 9 cm. The length of the third side may lie between 3 cm and 16 cm.
Explanation:
From the question, it is given that, the length of two sides of a triangle is 7 cm and 9 cm.
Let us assume the length of the third side of the triangle is ‘P’.
We know that, the sum of the two sides of the triangle is greater than the third side.
So, 7 + 9 > P
16 > P
Now, difference between two sides = 9 – 7 = 2
Therefore, the third side is greater than 2 and smaller than 16.
i.e. 3 cm and 16 cm
APPEARS IN
RELATED QUESTIONS
Is it possible to have a triangle with the following sides?
6 cm, 3 cm, 2 cm
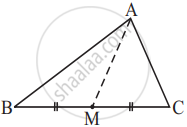
AM is a median of a triangle ABC.
Is AB + BC + CA > 2 AM?
(Consider the sides of triangles ΔABM and ΔAMC.)
ABCD is quadrilateral.
Is AB + BC + CD + DA > AC + BD?

The lengths of two sides of a triangle are 12 cm and 15 cm. Between what two measures should the length of the third side fall?
Angles Q and R of a ΔPQR are 25° and 65°.
Write which of the following is true:

The lengths of two sides of a triangle are 6 cm and 8 cm. Between which two numbers can the length of the third side fall?
In ∆PQR ______.
If length of two sides of a triangle are 6 cm and 10 cm, then the length of the third side can be ______.
Sum of two sides of a triangle is greater than or equal to the third side.
If the areas of two rectangles are same, they are congruent.
