Advertisements
Advertisements
Question
The perimeter of a triangle is 72cm and its sides are in the ratio 3:4:5. Find its area and the length of the altitude corresponding to the longest side.
Solution
Perimeter P of the triangle = P = 72cm
Ratio of the sides = 3:4:5
Let the constant of proportionality be k
⇒ 3k + 4k + 5k = 72
⇒ 12k = 72
⇒ k = `(72)/(12)`
= 6
∴ the sides are: 3 x 6, 4 x 6 and 5 x 6
I.e. 18cm, 24cm and 30cm
We know that, Area of a Triangle whose sides are a, b, and c and semiperimeter is s given by `sqrt("s"("s" - "a")("s" - "b")("s" - "c")); "s" = ("a" + "b" + "c")/(2)`
For a triangle whose sides are 18cm, 24cm and 30cm
i.e. a = 18 b = 24 and c = 30, s = `(72)/(2)` = 36
Area
= `sqrt(36(36 - 18)(36 - 24)(36 - 30)`
= `sqrt(36(18)(12)(6)`
= 21.6cm2
Let the length of the perpendicular of the triangle to the side 15cm be h cm i.e. height = h cm
We also know that, Area of a Triangle = `(1)/(2)"b.h" "i.e." (1)/(2)("Base" xx "Height")`
Area of a Traingle with base = 30cm and height = h cm
⇒ `(1)/(2)15."h"` = 216cm2
⇒ h = `(216 xx 2)/(30)`
= 14.4cm.
APPEARS IN
RELATED QUESTIONS
Find the area of an isosceles triangle with perimeter is 36 cm and the base is 16 cm.
Two sides of a triangle are 6 cm and 8 cm. If the height of the triangle corresponding to 6 cm side is 4 cm; find :
(i) area of the triangle
(ii) height of the triangle corresponding to 8 cm side.
The base and the height of a triangle are in the ratio 4: 5. If the area of the triangle is 40 m2; find its base and height.
The altitude and the base of a triangular field are in the ratio 6: 5. If its cost is ₹ 49,57,200 at the rate of ₹ 36,720 per hectare and 1 hectare = 10,000 sq. m, find (in metre) dimensions of the field.
The base of a triangle field and its height are in the ratio 2:5. If the cost of cultivating the field at Rs.42 per sq. m is Rs.7560, find its base and height.
The table given below contains some measures of the triangle. Find the unknown values.
| Side 1 | Side 2 | Side 3 | Perimeter |
| 6 cm | 5 cm | 2 cm | ? |
Find the perimeter of A scalene triangle with sides 7 m, 8 m, 10 m
A piece of wire is 36 cm long. What will be the length of each side if we form an equilateral triangle
The perimeter of a triangle is 28 cm. One of it’s sides is 8cm. Write all the sides of the possible isosceles triangles with these measurements.
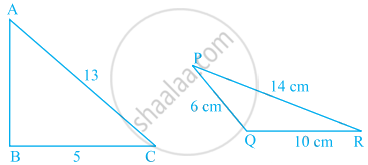
In the given figures, perimeter of ΔABC = perimeter of ΔPQR. Find the area of ΔABC.