Advertisements
Advertisements
Question
The point A divides the join of P (−5, 1) and Q(3, 5) in the ratio k:1. Find the two values of k for which the area of ΔABC where B is (1, 5) and C(7, −2) is equal to 2 units.
Solution
GIVEN: point A divides the line segment joining P (−5, 1) and Q (3, −5) in the ratio k: 1
Coordinates of point B (1, 5) and C (7, −2)
TO FIND: The value of k
PROOF: point A divides the line segment joining P (−5, 1) and Q (3, −5) in the ratio k: 1
So the coordinates of A are `(3k+1)/(k+1),(5k+1)/(k+1)`
We know area of triangle formed by three points (x1,y1)(x2,y2) and (x3,y3)s given by
Δ `= 1/2([x1y2+x2y3+x3y1)-(x2y1+x3y2+x1y3)]`
Now Area of ΔABC= 2 sq units.
Taking three points A `[(3k+1)/(k+1),(5k+1)/(k+1)]`, B (1, 5) and C (7, −2)
`2=1/2[{((3k-5)/(k+1))5-2+7((5k+1)/(k+1))}-((5k+1)/(k+1)) +35-2((3k-5)/(k-1))]`
`2=1/2[{((15k-25)/(k+1))-2+((35k+7)/(k+1))}-((5k-1)/(k+1))+35-((6k-10)/(k+1))]`
`2=1/2[((15k-25-2k+35k+7)/(k+1))-((5k+1+35k-6k+10)/(k+1))]`
`2=1/2[((48-20-(34k+46))/(k+1))]`
`2=1/2[((48k-20)-(34k+46))/(k+1))]`
`2=1/2[((14k-66)/(k+1))]`
`2=[((7k-33)/(k-1))]`
`+-=((7k-33)/(k+1))]`
⇒ 7k -33= ±2(k+1)
⇒ 7k -33= 2(k+1), 7k-33= -2(k+1)
⇒7k -33=2k +2, 7k+2k= +33- 2
⇒ 7k -2k=33 +2, 7k+2k =+33- 2
⇒ 5k =35,9k=31
`⇒k =35/5,k=31/9 `
⇒ k=7,k=`31/9`
Hence k=7 or `31/9`
APPEARS IN
RELATED QUESTIONS
Find a relation between x and y if the points (x, y), (1, 2) and (7, 0) are collinear.
Two vertices of a triangle are (1, 2), (3, 5) and its centroid is at the origin. Find the coordinates of the third vertex.
Prove that the points A(2, 4), b(2, 6) and (2 +`sqrt(3)` ,5) are the vertices of an equilateral triangle
For what value of k(k>0) is the area of the triangle with vertices (-2, 5), (k, -4) and (2k+1, 10) equal to 53 square units?
Find a relation between x and y, if the points A(2, 1), B(x, y) and C(7,5) are collinear.
Find the value of p for which the points (−5, 1), (1, p) and (4, −2) are collinear.
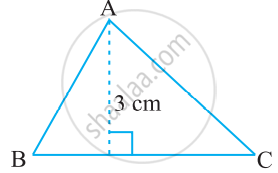
Find BC, if the area of the triangle ABC is 36 cm2 and the height AD is 3 cm.
The value of the determinant `abs((1,"x","x"^3),(1,"y","y"^3),(1,"z","z"^3))` is ____________.
If the points (2, -3), (k, -1), and (0, 4) are collinear, then find the value of 4k.
Points A(3, 1), B(12, –2) and C(0, 2) cannot be the vertices of a triangle.
