Advertisements
Advertisements
Question
The radii of the base of a cylinder and a cone are in the ratio 3 : 4 and their heights are in the ratio 2 : 3. What is the ratio of their volumes?
Solution
Let r1 and r2 be the radii of the base of a cylinder and a cone.
The volume of cylinder`V_1 = pir_1^2 h_1`…… (i)
The volume of cone `V_2 = pir_2^2 h_2`…… (ii)
Dividing (i) by (ii), the, we get
`V_2/v_2 = (pir_1^2 h_1)/(1/3pir_2^2 h_2)`
`(= 3 xx (r_1/r_2)^2 xx (h_1/h_2))/(((r_1/r_2 = 3/4 , h_1 /h_2 = 2/3, "given")))`
`V_1/V_2= 3 xx (3/4)^2 xx 2/3`
`V_1/V_2 = 9/8`
`V_1 : V_2 = 9:8`
APPEARS IN
RELATED QUESTIONS
A container, opened from the top and made up of a metal sheet, is in the form of a frustum of a cone of height 16 cm with radii of its lower and upper ends as 8 cm and 20 cm respectively. Find the cost of the milk which can completely fill the container, at the rate of Rs.20 per litre. Also find the cost of metal sheet used to make the container, if it costs Rs.8 per 100 cm2. [Take π = 3.14]
A frustum of a cone is 9 cm thick and the diameters of its circular ends are 28 cm and 4 cm. Find the volume and lateral surface area of the frustum.
(Take π = 22/7).
If the areas of circular bases of a frustum of a cone are 4 cm2 and 9 cm2 respectively and the height of the frustum is 12 cm. What is the volume of the frustum?
A cylinder and a cone are of the same base radius and of same height. Find the ratio of the value of the cylinder to that of the cone.
A reservoir is in the shape of a frustum of a right circular cone. It is 8 m across at the top and 4 m across at the bottom. If it is 6 m deep, then its capacity is
A hollow sphere of internal and external diameters 4 cm and 8 cm respectively is melted into a cone of base diameter 8 cm. The height of the cone is
The diameters of two circular ends of a bucket are 44 cm and 24 cm, and the height of the bucket is 35 cm. The capacity of the bucket is
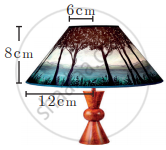
The frustum shaped outer portion of the table lamp has to be painted including the top part. Find the total cost of painting the lamp if the cost of painting 1 sq.cm is ₹ 2.
The slant height of a frustum of a cone is 4 m and the perimeter of circular ends is 18 m and 16 m. Find the cost of painting its curved surface area at ₹ 100 per sq. m
A metallic spherical shell of internal and external diameters 4 cm and 8 cm, respectively is melted and recast into the form a cone of base diameter 8 cm. The height of the cone is ______.
