Advertisements
Advertisements
Question
The radius of a circle with centre at origin is 30 units. Write the coordinates of the points where the circle intersects the axes. Find the distance between any two such points.
Solution
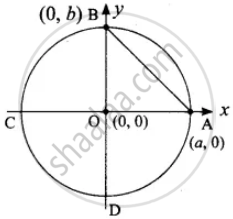
Radius of the circle = 30 units.
The point O is (0, 0).
Let a intersect the x-axis and b intersect the y-axis.
∴ The point A is (a, 0) and B is (0, b)
Distance = `sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2`
OA = `sqrt(("a" - 0)^2 + (0 - 0)^2`
30 = `sqrt("a"^2)`
Squaring on both sides
302 = a2
∴ a = 30
The point A is (30, 0)
OB = `sqrt((0 - 0)^2 + ("b" - 0)^2`
= `sqrt(0^2 + "b"^2)`
30 = `sqrt("b"^2)`
Squaring on both sides
302 = b2
∴ b = 30
The point B is (0, 30)
Distance AB = `sqrt((30 - 0)^2 + (0 - 30)^2`
= `sqrt(30^2 + 30^2)`
= `sqrt(900 + 900)`
= `sqrt(1800)`
= `sqrt(2 xx 900)`
= `30sqrt(2)`
∴ Distance between the two points = `30sqrt(2)`
APPEARS IN
RELATED QUESTIONS
If the co-ordinate of A is x and that of B is y, find d(A, B).
x = - 3, y = 7
On a number line, co-ordinates of P, Q, R are 3, -5 and 6 respectively. State with reason whether the following statement is true or false.
d(P, Q) + d(Q, R) = d(P, R)
Co-ordinates of the pair of points are given below. Hence find the distance between the pair.
-9, -1
Show that the following points taken in order to form an isosceles triangle
A(6, −4), B(−2, −4), C(2, 10)
Show that the following points taken in order to form an equilateral triangle
`"A"(sqrt(3), 2), "B"(0, 1), "C"(0, 3)`
Show that the following points taken in order to form the vertices of a parallelogram
A(−3, 1), B(−6, −7), C(3, −9) and D(6, −1)
Verify that the following points taken in order to form the vertices of a rhombus
A(1, 1), B(2, 1), C(2, 2) and D(1, 2)
If the points A(2, 0), B(– 6, 0), C(3, a – 3) lie on the x-axis then the value of a is _____
Find the distance with the help of the number line given below.

d(J, H)
Find the distance with the help of the number line given below.

d(O, E)
