Advertisements
Advertisements
Question
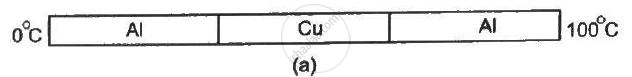
The three rods shown in figure have identical geometrical dimensions. Heat flows from the hot end at a rate of 40 W in the arrangement (a). Find the rates of heat flow when the rods are joined as in arrangement (b) and in (c). Thermal condcutivities of aluminium and copper are 200 W m−1°C−1 and 400 W m−1°C−1 respectively.
Solution
For arrangement (a),
Temperature of the hot end ,T1 = 100°C
Temperature of the cold end ,T2 = 0°C
`R_s = R_1 + R_2 + R_3`
`(l)/(K_{AI}A) + l/(K_{cu}A) + {l}/(K_{Al}A)`
`(l)/(A) ( 1/200 +1/400 + 1/200)`
`1/A (5/400)`
`1/Axx 1/80`
`(dQ)/d = q = Rate of flow of heat = (T_1 - T_2)/R_s `
`= (100 - 0)/((1/Axx1/(80))`
Given :
q = 40W
`40 = (100)/(1/Axx 1/80)`
`⇒ l/A = 200`
`⇒ l/A = 1/200 `
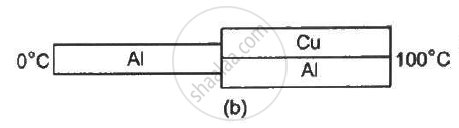
For arrangement (b),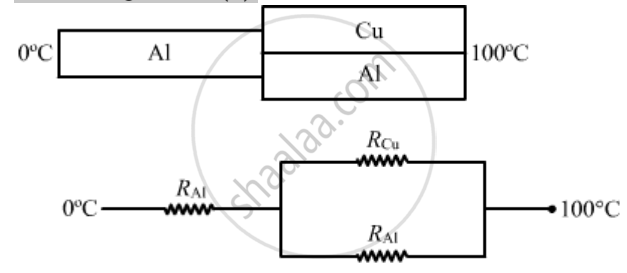
`"R_net" = R_[AI} + (R_cuxxR_{AI})/(R_cu = R_{AI)}`
`= l / (K_AI A) + (l/(K_cuA)xxl/K_(AI))/(l / (K_{cu}A9 )+ (l)/ (K_{AL}A)`
`= (l)/(A.K_Al) +( l )/ (A(K_AL + K_cu)) `
`= l/A (1/200 + 1/"200 + 400")`
`=l/A ( 1/200 + 1/600)`
= `4/600 1/A`
Rate of flow of heat is given by
`q = (T_1 - T_2)/ R_"net"`
`= ((100 - 0))/ (4 l) 600 A`
`= (100xx600)/4 xx1/200`
= 75 W
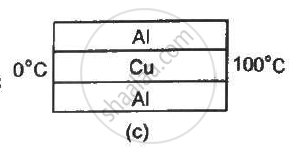
For arrangement (c),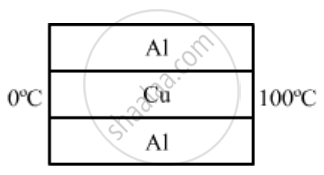
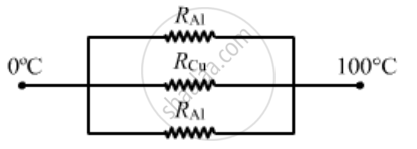
`1 / (R"net") = (1)/(R_{AI)) + 1/R_(cu)+ 1/(R_AI) `
`= K_{K_{AI}}/ (l)+ K_(cuA)/l + (K_{Al}A)/l`
`1/R_"net" = (K_Al + K_( cu) + K_(Al))A/l`
`l /R_"(net)" = (200 + 400 +200)`
`1/ R_"net" = 200/800`
`=(100)/(1/4)`
rate of heat flow = `(DeltaT)/R_"net"`
`= 100/1/4`400 W
APPEARS IN
RELATED QUESTIONS
A bullet of mass 20 g enters into a fixed wooden block with a speed of 40 m s−1 and stops in it. Find the change in internal energy during the process.
A brick weighing 4.0 kg is dropped into a 1.0 m deep river from a height of 2.0 m. Assuming that 80% of the gravitational potential energy is finally converted into thermal energy, find this thermal energy is calorie.
A block of mass 100 g slides on a rough horizontal surface. If the speed of the block decreases from 10 m s−1 to 5 m s−1, find the thermal energy developed in the process.
The blocks of masses 10 kg and 20 kg moving at speeds of 10 m s−1 and 20 m s−1respectively in opposite directions, approach each other and collide. If the collision is completely inelastic, find the thermal energy developed in the process.
The thermal conductivity of a rod depends on
One end of a metal rod is kept in a furnace. In steady state, the temperature of the rod
A pitcher with 1-mm thick porous walls contains 10 kg of water. Water comes to its outer surface and evaporates at the rate of 0.1 g s−1. The surface area of the pitcher (one side) = 200 cm2. The room temperature = 42°C, latent heat of vaporization = 2.27 × 106 J kg−1, and the thermal conductivity of the porous walls = 0.80 J s−1 m−1°C−1. Calculate the temperature of water in the pitcher when it attains a constant value.
Three rods of lengths 20 cm each and area of cross section 1 cm2 are joined to form a triangle ABC. The conductivities of the rods are KAB = 50 J s−1 m−1°C−1, KBC = 200 J s−1m−1°C−1 and KAC = 400 J s−1 m−1°C−1. The junctions A, B and C are maintained at 40°C, 80°C and 80°C respectively. Find the rate of heat flowing through the rods AB, AC and BC.
A hollow tube has a length l, inner radius R1 and outer radius R2. The material has a thermal conductivity K. Find the heat flowing through the walls of the tube if (a) the flat ends are maintained at temperature T1 and T2 (T2 > T1) (b) the inside of the tube is maintained at temperature T1 and the outside is maintained at T2.
A composite slab is prepared by pasting two plates of thickness L1 and L2 and thermal conductivites K1 and K2. The slabs have equal cross-sectional area. Find the equivalent conductivity of the composite slab.
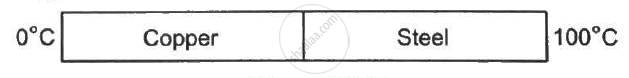
Figure (28-E2) shows a copper rod joined to a steel rod. The rods have equal length and equal cross sectional area. The free end of the copper rod is kept at 0°C and that of the steel rod is kept at 100°C. Find the temperature at the junction of the rods. Conductivity of copper = 390 W m−1°C−1 and that if steel = 46 W m−1°C−1.
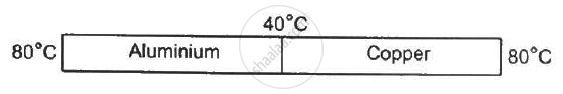
Following Figure shows an aluminium rod joined to a copper rod. Each of the rods has a length of 20 cm and area of cross section 0.20 cm2. The junction is maintained at a constant temperature 40°C and the two ends are maintained at 80°C. Calculate the amount of heat taken out from the cold junction in one minute after the steady state is reached. The conductivites are KAt = 200 W m−1°C−1 and KCu = 400 W m−1°C−1.
Suppose the bent part of the frame of the previous problem has a thermal conductivity of 780 J s−1 m−1 °C−1 whereas it is 390 J s−1 m−1°C−1 for the straight part. Calculate the ratio of the rate of heat flow through the bent part to the rate of heat flow through the straight part.
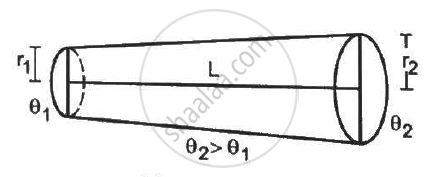
Find the rate of heat flow through a cross section of the rod shown in figure (28-E10) (θ2 > θ1). Thermal conductivity of the material of the rod is K.
Two bodies of masses m1 and m2 and specific heat capacities s1 and s2 are connected by a rod of length l, cross-sectional area A, thermal conductivity K and negligible heat capacity. The whole system is thermally insulated. At time t = 0, the temperature of the first body is T1 and the temperature of the second body is T2 (T2 > T1). Find the temperature difference between the two bodies at time t.
