Advertisements
Advertisements
Question
Three metallic cubes whose edges are 3 cm, 4 cm and 5 cm, are melted and recast into a single large cube. Find the edge of the new cube formed.
Solution
We have,
Edges of the cubes : a1 = 3 cm, a2 = 4 cm and a3 = 5 cm
Let the edge of the new cube be a.
Now,
` "Volume of the new cube" = a_1^3 +a_2^3+a_3^3`
`=>a^3 = 3^3 + 4^3 + 5^3`
⇒ a3 = 27 + 64 + 125
⇒ a3 = 216
`⇒ a = root(3)(216)`
∴ a = 6 cm
So, the edge of the new cube so formed is 6 cm.
APPEARS IN
RELATED QUESTIONS
A spherical glass vessel has a cylindrical neck 8 cm long, 2cm in diameter; the diameter of the spherical part is 8.5 cm. By measuring the amount of water it holds, a child finds its volume to be 345 cm3. Check whether she is correct, taking the above as the inside measurements, and π = 3.14
A heap of rice is in the form of a cone of base diameter 24 m and height 3.5 m. Find the volume of the rice. How much is canvas cloth required to just cover the heap?
A well of diameter 2m is dug14m deep. The earth taken out of it is spread evenly all around it to form an embankment of height 40cm. Find width of the embankment?
A well with inner radius 4m is dug 14m deep earth taken out of it has been spread evenly all around a width of 3m it to form an embankment. Find the height of the embankment?
A circus tent has cylindrical shape surmounted by a conical roof. The radius of the cylindrical base is 20 m. The heights of the cylindrical and conical portions are 4.2 m and 2.1 m respectively. Find the volume of the tent.
A cubical ice-cream brick of edge 22 cm is to be distributed among some children by filling ice-cream cones of radius 2 cm and height 7 cm up to the brim. How many children will get the ice-cream cones?
The figure shows a running track surrounding a grassed enclosure PQRSTU. The enclosure consists of a rectangle PQST with a semicircular region at each end, PQ = 200 m; PT = 70 meters.
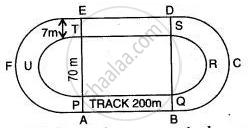
(i) Calculate the area of the grassed enclosure in m2.
(ii) Given that the track is of constant width 7 m, calculate the outer perimeter ABCDEF of the track. (Take π = `22/7`)
The volumes of two spheres are in the ratio 27 : 8. The ratio of their curved surface is ______.
The diameters of the two circular ends of the bucket are 44 cm and 24 cm. The height of the bucket is 35 cm. The capacity of the bucket is ______.
A surahi is the combination of ______.
