Advertisements
Advertisements
Question
Two parallel lines are cut by a transversal. For each pair of interior angles on the same side of the transversal, if one angle exceeds the twice of the other angle by 48°. Find the angles
Solution
Let the two parallel lines be m and n and l be the transversal
Let one of the interior angles on the same side of the transversal be x
Then the other will be 2x + 48°
We know that they are supplementary.
∴ x + (2x + 48°) = 180°
x + 2x + 48° = 180°
3x + 48° = 180°
3x + 48° − 48° = 180° − 48°
3x = 132°
x = `(132^circ)/3`
x = 44°
∴ One angle is x = 44°
Other angel is 2x + 48° = 2(44) + 48°
= 88 + 48°
= 136°
∴ The angles are 44° and 136°
APPEARS IN
RELATED QUESTIONS
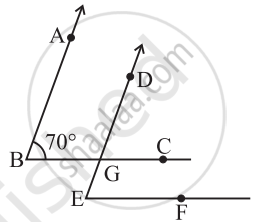
In the given figure, the arms of two angles are parallel.
If ∠ABC = 70°, then find
- ∠DGC
- ∠DEF
Find the measure of angle x in the following figure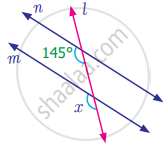
Find the measure of angle z in the following figure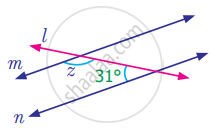
Find the value of angle a in the following figure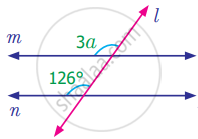
In the parking lot shown, the lines that mark the width space are parallel. If ∠1 =(x + 39)°, ∠2 = (2x – 3y)° find x and y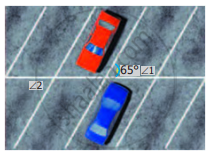
In the given figure, line l intersects two parallel lines PQ and RS. Then, which one of the following is not true?

Interior angles on the same side of a transversal with two distinct parallel lines are complementary angles.
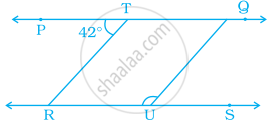
In the given figure, PQ || RS, TR || QU and ∠PTR = 42°. Find ∠QUR.
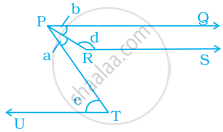
In the given figure, PQ, RS and UT are parallel lines. If c = 57° and a = `c/3`, find the value of d.
In the given figure, two parallel lines l and m are cut by two transversals p and q. Determine the values of x and y.

