Advertisements
Advertisements
Question
Ultraviolet light of wavelength 2271 Å from a 100 W mercury source irradiates a photo-cell made of molybdenum metal. If the stopping potential is −1.3 V, estimate the work function of the metal. How would the photo-cell respond to a high intensity (∼105 W m−2) red light of wavelength 6328 Å produced by a He-Ne laser?
Solution
Wavelength of ultraviolet light, λ = 2271 Å = 2271 × 10−10 m
Stopping potential of the metal, V0 = 1.3 V
Planck’s constant, h = 6.6 × 10−34 J
Charge on an electron, e = 1.6 × 10−19 C
Work function of the metal = `phi_0`
Frequency of light = v
We have the photo-energy relation from the photoelectric effect as:
`phi_0` = hv − eV0
= `"hc"/lambda - "eV"_0`
= `(6.6 xx 10^(-34) xx 3 xx 10^8)/(2271 xx 10^(-10)) - 1.6 xx 10^(-19) xx 1.3`
= `8.72 xx 10^(-19) - 2.08 xx 10^(-19)`
= `6.64 xx 10^(-19) "J"`
= `(6.64 xx 10^(-19))/(1.6 xx 10^(-19))`
= 4.15 eV
Let v0 be the threshold frequency of the metal.
∴ `phi_0 = "hv"_0`
`"v"_0 = phi_0/"h"`
= `(6.64 xx 10^(-19))/(6.6 xx 10^(-34))`
= 1.006 × 1015 Hz
Wavelength of red light, `lambda_"r"` = 6328 Å = 6328 × 10−10 m
∴ Frequency of red light, `"v"_"r" = "c"/lambda_"r"`
= `(3 xx 10^8)/(6328 xx 10^(-10))`
= 4.74 × 1014 Hz
Since v0 > vr, the photocell will not respond to the red light produced by the laser.
APPEARS IN
RELATED QUESTIONS
Define the term 'intensity of radiation' in terms of photon picture of light.
Every metal has a definite work function. Why do all photoelectrons not come out with the same energy if incident radiation is monochromatic? Why is there an energy distribution of photoelectrons?
The following graph shows the variation of photocurrent for a photosensitive metal :
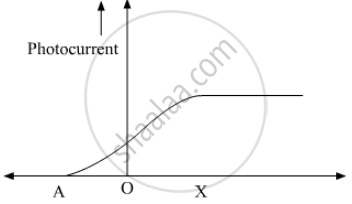
(a) Identify the variable X on the horizontal axis.
(b) What does the point A on the horizontal axis represent?
(c) Draw this graph for three different values of frequencies of incident radiation v1, v2 and v3 (v1 > v2 > v3) for same intensity.
(d) Draw this graph for three different values of intensities of incident radiation I1, I2 and I3 (I1 > I2 > I3) having same frequency.
What is the speed of a photon with respect to another photon if (a) the two photons are going in the same direction and (b) they are going in opposite directions?
Can a photon be deflected by an electric field? Or by a magnetic field?
Planck's constant has the same dimensions as
Let nr and nb be the number of photons emitted by a red bulb and a blue bulb, respectively, of equal power in a given time.
A point source of light is used in a photoelectric effect. If the source is removed farther from the emitting metal, the stopping potential
Photoelectric effect supports quantum nature of light because
(a) there is a minimum frequency below which no photoelectrons are emitted
(b) the maximum kinetic energy of photoelectrons depends only on the frequency of light and not on its intensity
(c) even when the metal surface is faintly illuminated the photoelectrons leave the surface immediately
(d) electric charge of the photoelectrons is quantised
A photon of energy hv is absorbed by a free electron of a metal with work-function hv − φ.
In which of the following situations, the heavier of the two particles has smaller de Broglie wavelength? The two particles
(a) move with the same speed
(b) move with the same linear momentum
(c) move with the same kinetic energy
(d) have fallen through the same height
Calculate the momentum of a photon of light of wavelength 500 nm.
(Use h = 6.63 × 10-34J-s = 4.14 × 10-15 eV-s, c = 3 × 108 m/s and me = 9.1 × 10-31kg)
Calculate the number of photons emitted per second by a 10 W sodium vapour lamp. Assume that 60% of the consumed energy is converted into light. Wavelength of sodium light = 590 nm
(Use h = 6.63 × 10-34J-s = 4.14 × 10-15 eV-s, c = 3 × 108 m/s and me = 9.1 × 10-31kg)
A parallel beam of monochromatic light of wavelength 663 nm is incident on a totally reflecting plane mirror. The angle of incidence is 60° and the number of photons striking the mirror per second is 1.0 × 1019. Calculate the force exerted by the light beam on the mirror.
(Use h = 6.63 × 10-34J-s = 4.14 × 10-15 eV-s, c = 3 × 108 m/s and me = 9.1 × 10-31kg)
The electric field associated with a light wave is given by `E = E_0 sin [(1.57 xx 10^7 "m"^-1)(x - ct)]`. Find the stopping potential when this light is used in an experiment on photoelectric effect with the emitter having work function 1.9 eV.
Consider a metal exposed to light of wavelength 600 nm. The maximum energy of the electron doubles when light of wavelength 400 nm is used. Find the work function in eV.
Consider a thin target (10–2 cm square, 10–3 m thickness) of sodium, which produces a photocurrent of 100 µA when a light of intensity 100W/m2 (λ = 660 nm) falls on it. Find the probability that a photoelectron is produced when a photons strikes a sodium atom. [Take density of Na = 0.97 kg/m3].
- Assertion (A): For the radiation of a frequency greater than the threshold frequency, the photoelectric current is proportional to the intensity of the radiation.
- Reason (R): Greater the number of energy quanta available, the greater the number of electrons absorbing the energy quanta and the greater the number of electrons coming out of the metal.
