Advertisements
Advertisements
Question
Using a free body diagram, show that it is easy to pull an object than to push it.
Solution
When a body is pushed at an arbitrary angle θ [0 to `π/2`], the applied force F can be resolved into two components as F sin 0 parallel to the surface and F cos 0 perpendicular to the surface as shown in the figure. The total downward force acting on the body is mg + F cos θ. It implies that the normal force acting on the body increases. Since there is no acceleration along the vertical direction the normal force N is equal to
Npush = mg + F cos θ …………(1)
As a result the maximal static friction also increases and is equal to
`f_S^max = mu_rN_{push} = mu_s("mg" + F cos theta)` .................(2)
Equation (2) shows that a greater force needs to be applied to push the object into motion.
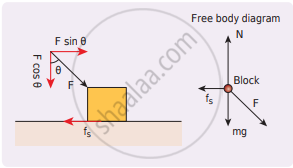
An object is pushed at an angle θ
When an object is pulled at an angle θ, the applied force is resolved into two components as shown in the figure. The total downward force acting on the object is –
Npull = mg – F cos θ ………….(3)
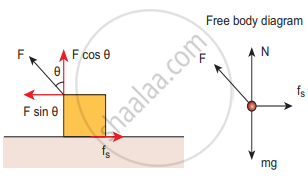
An object is pulled at an angle θ
Equation (3) shows that the normal force is less than – Npush. From equations (1) and (3), it is easier to pull an object than to push to make it move.
APPEARS IN
RELATED QUESTIONS
An object of mass m held against a vertical wall by applying horizontal force F as shown in the figure. The minimum value of the force F is ______

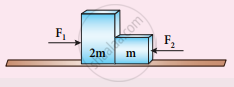
Two blocks of masses m and 2m are placed on a smooth horizontal surface as shown. In the first case, only a force F1 is applied from the left. Later only a force F2 is applied from the right. If the force acting at the interface of the two blocks in the two cases is the same, then F1 : F2 is ______
When an object is at rest on the inclined rough surface, ______
Apply Lami’s theorem on the slingshot and calculate the tension in each string?

The physics books are stacked on each other in the sequence: +1 volumes 1 and 2; +2 volumes 1 and 2 on a table.
a) Identify the forces acting on each book and draw the free body diagram.
b) Identify the forces exerted by each book on the other.
What are concurrent forces?
State Lami’s theorem.
