Advertisements
Advertisements
Question
Why does a galvanometer when connected in series with a capacitor show a momentary deflection, when it is being charged or discharged?
How does this observation lead to modifying the Ampere's circuital law?
Hence write the generalised expression of Ampere's law.
Solution
During charging and discharging of the capacitor, there is a flow of charge from the battery towards the plates of the capacitor, which produces a conduction current in the circuit. Hence, the galvanometer present in the circuit shows momentary deflection.
As the charge on the capacitor grows, the conduction current in the wires increases. When the capacitor is fully charged, the conduction current stops flowing in the wires. During charging or discharging of the capacitor, there is no conduction current between the plates of capacitor, as they are not connected by any conduction material. Therefore, in this region, a current must exist that makes the total current in the circuit continuous. This observation leads to modifying the Ampere's circuital law.
Thus, there must be displacement current along with the conduction current in the circuit having the property of continuity, although individually they may not be continuous.
Maxwell modified Ampere's circuital law in order to make it logically consistent. He stated Ampere's circuital law in the form,
This is the generalised expression of Ampere's law.
APPEARS IN
RELATED QUESTIONS
A rectangular coil of a moving coil galvanometer contains 50 turns each having area 12 cm2 . It is suspended in radial magnetic field 0.025 Wb/m2 by a fibre of twist constant 15 x10-10 Nm/degree. Calculate the sensitivity of the moving coil galvanometer.
- A circular coil of 30 turns and radius 8.0 cm carrying a current of 6.0 A is suspended vertically in a uniform horizontal magnetic field of magnitude 1.0 T. The field lines make an angle of 60° with the normal of the coil. Calculate the magnitude of the counter torque that must be applied to prevent the coil from turning.
- Would your answer change, if the circular coil in (a) were replaced by a planar coil of some irregular shape that encloses the same area? (All other particulars are also unaltered.)
Define the current sensitivity of a galvanometer ?
Explain the significance of a radial magnetic field when a current-carrying coil is kept in it.
A galvanometer coil has a resistance of 12 Ω and the metre shows full scale deflection for a current of 3 mA. How will you convert the metre into a voltmeter of range 0 to 18 V?
The coil of a moving coil galvanometer is wound over a metal frame in order to ______.
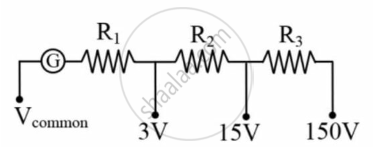
A voltmeter of variable ranges 3 V, 15 V, 150 V is to be designed by connecting resistances R1, R2, R3 in series with a galvanometer of resistance G = 20 Ω, as shown in Fig. The galvanometer gives full pass through its coil for 1 mA current i.e. "gives full pass through it's coil for 1 mA current". Then, the resistances R1, R2 and R3 (in kilo ohms) should be, respectively:
A galvanometer coil bas 500 turns and each tum has an average area of 3 × 10-4 m2. If a torque of 1.5 Nm is required to keep this coil parallel to a magnetic field when a current of 0.5 A is flowing through it, the strength of the field (in T) is ______.
A resistance of 3Ω is connected in parallel to a galvanometer of resistance 297Ω. Find the fraction of current passing through the galvanometer.
A galvanometer of resistance 100 Ω gives a full-scale deflection for a potential difference of 200 mV.
- What must be the resistance connected to convert the galvanometer into an ammeter of the range 0-200 mA?
- Determine resistance of the ammeter.
