Topics
Angle and Its Measurement
- Directed Angle
- Angles of Different Measurements
- Angles in Standard Position
- Measures of Angles
- Area of a Sector of a Circle
- Length of an Arc of a Circle
Trigonometry - 1
- Introduction of Trigonometry
- Trigonometric Functions with the Help of a Circle
- Signs of Trigonometric Functions in Different Quadrants
- Range of Cosθ and Sinθ
- Trigonometric Functions of Specific Angles
- Trigonometric Functions of Negative Angles
- Fundamental Identities
- Periodicity of Trigonometric Functions
- Domain and Range of Trigonometric Functions
- Graphs of Trigonometric Functions
- Polar Co-ordinate System
Trigonometry - 2
- Trigonometric Functions of Sum and Difference of Angles
- Trigonometric Functions of Allied Angels
- Trigonometric Functions of Multiple Angles
- Trigonometric Functions of Double Angles
- Trigonometric Functions of Triple Angle
- Factorization Formulae
- Formulae for Conversion of Sum Or Difference into Product
- Formulae for Conversion of Product in to Sum Or Difference
- Trigonometric Functions of Angles of a Triangle
Determinants and Matrices
- Definition and Expansion of Determinants
- Minors and Cofactors of Elements of Determinants
- Properties of Determinants
- Application of Determinants
- Determinant method
- Consistency of Three Equations in Two Variables
- Area of Triangle and Collinearity of Three Points
- Introduction of Matrices
- Types of Matrices
- Algebra of Matrices
- Properties of Matrix Multiplication
- Properties of Transpose of a Matrix
Straight Line
- Locus of a Points in a Co-ordinate Plane
- Straight Lines
- Equations of Line in Different Forms
- General Form of Equation of a Line
- Family of Lines
Circle
- Different Forms of Equation of a Circle
- General Equation of a Circle
- Parametric Form of a Circle
- Tangent
- Condition of tangency
- Tangents from a Point to the Circle
- Director circle
Conic Sections
- Double Cone
- Conic Sections
- Parabola
- Ellipse
- Hyperbola
Measures of Dispersion
- Meaning and Definition of Dispersion
- Measures of Dispersion
- Range of Data
- Variance
- Standard Deviation
- Change of Origin and Scale of Variance and Standard Deviation
- Standard Deviation for Combined Data
- Coefficient of Variation
Probability
- Basic Terminologies
- Event and Its Types
- Concept of Probability
- Addition Theorem for Two Events
- Conditional Probability
- Multiplication Theorem on Probability
- Independent Events
- Bayes’ Theorem
- Odds (Ratio of Two Complementary Probabilities)
Complex Numbers
- Introduction of Complex Number
- Concept of Complex Numbers
- Algebraic Operations of Complex Numbers
- Square Root of a Complex Number
- Fundamental Theorem of Algebra
- Argand Diagram Or Complex Plane
- De Moivres Theorem
- Cube Root of Unity
- Set of Points in Complex Plane
Sequences and Series
- Concept of Sequences
- Arithmetic Progression (A.P.)
- Geometric Progression (G. P.)
- Harmonic Progression (H. P.)
- Arithmetico Geometric Series
- Power Series
Permutations and Combination
- Fundamental Principles of Counting
- Invariance Principle
- Factorial Notation
- Permutations
- Permutations When All Objects Are Distinct
- Permutations When Repetitions Are Allowed
- Permutations When Some Objects Are Identical
- Circular Permutations
- Properties of Permutations
- Combination
- Properties of Combinations
Methods of Induction and Binomial Theorem
- Principle of Mathematical Induction
- Binomial Theorem for Positive Integral Index
- General Term in Expansion of (a + b)n
- Middle term(s) in the expansion of (a + b)n
- Binomial Theorem for Negative Index Or Fraction
- Binomial Coefficients
Sets and Relations
- Sets and Their Representations
- Types of Sets
- Operations on Sets
- Intervals
- Concept of Relation
Functions
- Concept of Functions
- Algebra of Functions
Limits
- Concept of Limits
- Factorization Method
- Rationalization Method
- Limits of Trigonometric Functions
- Substitution Method
- Limits of Exponential and Logarithmic Functions
- Limit at Infinity
Continuity
- Continuous and Discontinuous Functions
Differentiation
- Definition of Derivative and Differentiability
- Rules of Differentiation (Without Proof)
- Derivative of Algebraic Functions
- Derivatives of Trigonometric Functions
- Derivative of Logarithmic Functions
- Derivatives of Exponential Functions
- L' Hospital'S Theorem
- Definition of Relation
- Domain
- Co-domain and Range of a Relation
Definition
A relation R from a non-empty set A to a non-empty set B is a subset of the cartesian product A × B. The subset is derived by describing a relationship between the first element and the second element of the ordered pairs in A × B. The second element is called the image of the first element.
The set of all first elements of the ordered pairs in a relation R from a set A to a set B is called the domain of the relation R.
The set of all second elements in a relation R from a set A to a set B is called the range of the relation R. The whole set B is called the codomain of the relation R. Note that range ⊂ codomain.
Notes
(i) A relation may be represented algebraically either by the Roster method or by the Set-builder method.
(ii) An arrow diagram is a visual representation of a relation.
Consider the two sets P = {a, b, c} and Q = {Ali, Bhanu, Binoy, Chandra, Divya}.
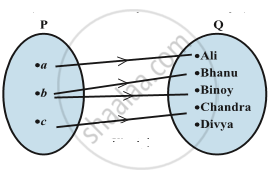
The cartesian product of P and Q has 15 ordered pairs which can be listed as P × Q = {(a, Ali), (a,Bhanu), (a, Binoy), ..., (c, Divya)}. We can now obtain a subset of P × Q by introducing a relation R between the first element x and the second element y of each ordered pair (x, y) as R= { (x,y): x is the first letter of the name y, x ∈ P, y ∈ Q}. Then R = {(a, Ali), (b, Bhanu), (b, Binoy), (c, Chandra)} A visual representation of this relation R (called an arrow diagram) is shown in Fig .
A relation R from A to A is also stated as a relation on A.
