Advertisements
Advertisements
प्रश्न
A capacitor of capacitance 12.0 μF is connected to a battery of emf 6.00 V and internal resistance 1.00 Ω through resistanceless leads. 12.0 μs after the connections are made, what will be (a) the current in the circuit (b) the power delivered by the battery (c) the power dissipated in heat and (d) the rate at which the energy stored in the capacitor is increasing?
Advertisements
उत्तर
Given,
Capacitance of capacitor, C= 12.0 μF = 12 × 10−6 F
Emf of battery, V0 = 6.00 V
Internal resistance of battery, R = 1 Ω
Time interval, t = 12 μs
(a) Charging current in the circuit is given as,
i = i0e−t/RC
Current at, t = 12.0 μs
\[i = \frac{V_0}{R} e^{- t/RC} \]
\[i = \frac{6}{1} \times e^{- 1} \]
\[i = 2 . 207 = 2 . 21 A\]
(b) During charging, charge on the capacitor at any time ''t'' is given as
\[Q = C V_0 (1 - e^{- \frac{t}{RC}} )\]
Work done by battery in in time delivering this charge is,
W = QV0
Power deliver by the battery in time ''t'' is,
\[P = \frac{C {V_0}^2 (1 - e^{- \frac{t}{RC}} )}{t}\]
Putting, t = 12 μs
\[P = \frac{12 \times {10}^{- 6} {V_0}^2 (1 - e^{- 1} )}{12 \times {10}^{- 6}}\]
\[ \Rightarrow P = 13 . 25 W\]
(c) Energy stroed in the capacitor at any instant of time is given as,
\[U = \frac{1}{2}\frac{Q^2}{C}\]
\[ \Rightarrow U = \frac{1}{2}\frac{C^2 {V_0}^2 (1 - e^{- \frac{t}{RC}} )^2}{C}\]
\[ \Rightarrow U = \frac{1}{2}C {V_0}^2 (1 - e^{- \frac{t}{RC}} )^2\]
Rate at which the energy stored in the capacitor is,
\[\frac{dU}{dt} = \frac{1}{2}C {V_0}^2 \times 2(1 - e^{- \frac{t}{RC}} ) \times ( e^{- \frac{t}{RC}} ) \times \frac{1}{RC}\]
\[\Rightarrow \frac{dU}{dt} = \frac{{V_0}^2}{R}( e^{- \frac{t}{RC}} - e^{- \frac{2t}{RC}} )\]
\[ \Rightarrow \frac{dU}{dt} = \frac{6 \times 6}{1}( e^{- 1} - e^{- 2} )\]
\[ \Rightarrow \frac{dU}{dt} = 8 . 37 W\]
So, the power dissipated in heat = \[P - \frac{dU}{dt}= 13.25-8.37 = 4.87 W\]
(d) Rate at which the energy stored in the capacitor is increasing
\[\Rightarrow \frac{dU}{dt} = 8 . 37 W\]
APPEARS IN
संबंधित प्रश्न
Find the charge on the capacitor as shown in the circuit.
Find the ratio of energy stored in the two configurations if they are both connected to the same source.
The energy density in the electric field created by a point charge falls off with the distance from the point charge as
A capacitor of capacitance 500 μF is connected to a battery through a 10 kΩ resistor. The charge stored in the capacitor in the first 5 s is larger than the charge stored in the next.
(a) 5 s
(b) 50 s
(c) 500 s
(d) 500 s
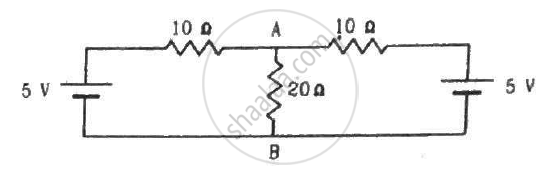
(a) Find the current in the 20 Ω resistor shown in the figure. (b) If a capacitor of capacitance 4 μF is joined between the points A and B, what would be the electrostatic energy stored in it in steady state?
A 100 μF capacitor is joined to a 24 V battery through a 1.0 MΩ resistor. Plot qualitative graphs (a) between current and time for the first 10 minutes and (b) between charge and time for the same period.
How many time constants will elapse before the charge on a capacitors falls to 0.1% of its maximum value in a discharging RC circuit?
How many time constants will elapse before the energy stored in the capacitor reaches half of its equilibrium value in a charging RC circuit?
Two capacitors of capacitances 4⋅0 µF and 6⋅0 µF are connected in series with a battery of 20 V. Find the energy supplied by the battery.
A capacitor of capacitance C is connected to a battery of emf ε at t = 0 through a resistance R. Find the maximum rate at which energy is stored in the capacitor. When does the rate have this maximum value?
Each capacitor in figure has a capacitance of 10 µF. The emf of the battery is 100 V. Find the energy stored in each of the four capacitors.
A capacitor with stored energy 4⋅0 J is connected with an identical capacitor with no electric field in between. Find the total energy stored in the two capacitors.
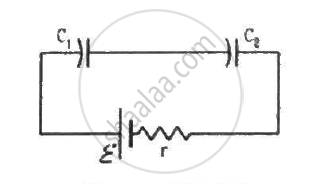
Consider the situation shown in figure. The switch is closed at t = 0 when the capacitors are uncharged. Find the charge on the capacitor C1 as a function of time t.
A capacitor of capacitance C is given a charge Q. At t = 0, it is connected to an ideal battery of emf ε through a resistance R. Find the charge on the capacitor at time t.
A metal sphere of radius R is charged to a potential V.
- Find the electrostatic energy stored in the electric field within a concentric sphere of radius 2 R.
- Show that the electrostatic field energy stored outside the sphere of radius 2 R equals that stored within it.
A capacitor is charged by a battery and energy stored is 'U'. Now the battery is removed and the distance between plates is increased to four times. The energy stored becomes ______.
An air-filled parallel plate capacitor has a uniform electric field `overset(->)("E")` in the space between the plates. If the distance between the plates is 'd' and the area of each plate is 'A', the energy stored in the capacitor is ______
(∈0 = permittivity of free space)
A parallel plate capacitor has a uniform electric field ‘`vec "E"`’ in the space between the plates. If the distance between the plates is ‘d’ and the area of each plate is ‘A’, the energy stored in the capacitor is ______
(ε0 = permittivity of free space)
Prove that, if an insulated, uncharged conductor is placed near a charged conductor and no other conductors are present, the uncharged body must be intermediate in potential between that of the charged body and that of infinity.
In a capacitor of capacitance 20 µF, the distance between the plates is 2 mm. If a dielectric slab of width 1 mm and dielectric constant 2 is inserted between the plates, what is the new capacitance?
