Advertisements
Advertisements
प्रश्न
A child stands at the centre of a turntable with his two arms outstretched. The turntable is set rotating with an angular speed of 40 rev/min. How much is the angular speed of the child if he folds his hands back and thereby reduces his moment of inertia to 2/5 times the initial value? Assume that the turntable rotates without friction.
उत्तर १
Suppose, initial moment of inertia of the child is I1 Then final moment of inertia,
`I_2 = 2/3 I_l`
Also `v_1 = 40 " rev min"^(-I)`
By using the principle of conversion of angular, momentum, we get
`I_1omega_1 = I_2omega_2 or I_1(2piv_1) = I_2(2piv_2)`
or `v_2 = (I_1v_1)/I_2 = (I_1xx40)/(2/5 xx I_1) = 100 rev min^(-1)`
उत्तर २
100 rev/min
Initial angular velocity, ω1= 40 rev/min
Final angular velocity = ω2
The moment of inertia of the boy with stretched hands = I1
The moment of inertia of the boy with folded hands = I2
The two moments of inertia are related as:
`I_2 = 2/5 I_1`
Since no external force acts on the boy, the angular momentum L is a constant.
Hence, for the two situations, we can write:
`I_2omega_2 = I_1omega_1`
`omega_2=I_1/I_2 omega_1`
`= I_1/(2/5 I_1) xx 40 = 5/2 xx 40`
= 100 rev/min
APPEARS IN
संबंधित प्रश्न
Find the moment of inertia of a sphere about a tangent to the sphere, given the moment of inertia of the sphere about any of its diameters to be 2MR2/5, where M is the mass of the sphere and R is the radius of the sphere.
Given the moment of inertia of a disc of mass M and radius R about any of its diameters to be MR2/4, find its moment of inertia about an axis normal to the disc and passing through a point on its edge
Torques of equal magnitude are applied to a hollow cylinder and a solid sphere, both having the same mass and radius. The cylinder is free to rotate about its standard axis of symmetry, and the sphere is free to rotate about an axis passing through its centre. Which of the two will acquire a greater angular speed after a given time?
A hoop of radius 2 m weighs 100 kg. It rolls along a horizontal floor so that its centre of mass has a speed of 20 cm/s. How much work has to be done to stop it?
A string is wrapped on a wheel of moment of inertia 0⋅20 kg-m2 and radius 10 cm and goes through a light pulley to support a block of mass 2⋅0 kg as shown in the following figure. Find the acceleration of the block.
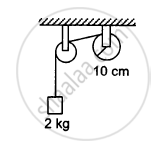
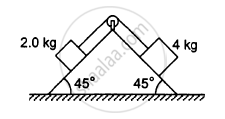
The pulley shown in the following figure has a radius 10 cm and moment of inertia 0⋅5 kg-m2about its axis. Assuming the inclined planes to be frictionless, calculate the acceleration of the 4⋅0 kg block.
A wheel of moment of inertia 0⋅10 kg-m2 is rotating about a shaft at an angular speed of 160 rev/minute. A second wheel is set into rotation at 300 rev/minute and is coupled to the same shaft so that both the wheels finally rotate with a common angular speed of 200 rev/minute. Find the moment of inertia of the second wheel.
From a circular ring of mass, ‘M’ and radius ‘R’ an arc corresponding to a 90° sector is removed. The moment of inertia of the remaining part of the ring about an axis passing through the centre of the ring and perpendicular to the plane of the ring is ‘K’ times ‘MR2’. Then the value of ‘K’ is ______.
Why does a solid sphere have smaller moment of inertia than a hollow cylinder of same mass and radius, about an axis passing through their axes of symmetry?
Consider a badminton racket with length scales as shown in the figure.
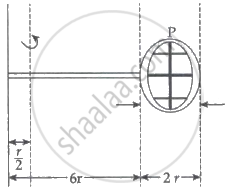
If the mass of the linear and circular portions of the badminton racket is the same (M) and the mass of the threads is negligible, the moment of inertia of the racket about an axis perpendicular to the handle and in the plane of the ring at, `r/2` distance from the ends A of the handle will be ______ Mr2.
