Advertisements
Advertisements
प्रश्न
A cylindrical vessel of diameter 12 cm contains 800π cm3 of water. A cylindrical glass piece of diameter 8.0 cm and height 8.0 cm is placed in the vessel. If the bottom of the vessel under the glass piece is seen by the paraxial rays (see figure), locate its image. The index of refraction of glass is 1.50 and that of water is 1.33.

उत्तर
Given,
Diameter of the cylindrical vessel = 12 cm
∴ radius r = 6 cm
Diameter of the cylindrical glass piece = 8 cm
∴ radius r' = 4 cm and its height, h1 = 8 cm
Refractive index of glass, μg = 1.5
Refractive index of water, μw = 1.3
Let h be the final height of the water column.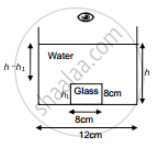
The volume of the cylindrical water column after the glass piece is put will be:
\[\pi\] r2h = 800π +
\[\pi\] r'2h1
or r2h = 800 + r'2h1
or (6)2 h = 800 + (4)2 8
\[or `h = \frac{800 + 128} ( 36 ) = 928/36 = 25.7 cm`
There will be two shifts; due to the glass block as well as water:
\[∆ t_1 = \left[ 1 - \frac{1}{\mu_g} \right] t_g = \left[ 1 - \frac{1}{\frac{3}{2}} \right] \times 8 = 2 . 26 cm\]
\[ ∆ t_2 = \left[ 1 - \frac{1}{\mu_w} \right] t_w = \left[ 1 - \frac{1}{\frac{4}{3}} \right] \times \left( 25 . 7 - 8 \right) = 4 . 44 cm\]
Hence, the total shift = (Δt1+ Δt2) = (2.66 + 4.44) cm = 7.1 cm above the bottom.
APPEARS IN
संबंधित प्रश्न
Use the mirror equation to deduce that the virtual image produced by a convex mirror is always diminished in size and is located between the focus and the pole.
Using mirror formula, explain why does a convex mirror always produce a virtual image.
An object is kept on the principal axis of a concave mirror of focal length 10 cm. at a distance of 15
cm from its pole. The image formed by the mirror is:
(a) Virtual and magnified
(b) Virtual and diminished
(c) Real and magnified
(d) Real and diminished
A point source of light is placed in front of a plane mirror.
following Figure shows two rays A and B being reflected by a mirror and going as A' and B'. The mirror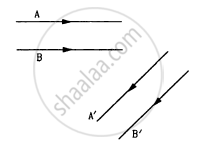
Mark the correct options.
Which of the following (referred to a spherical mirror) do (does) not depend on whether the rays are paraxial or not?
(a) Pole
(b) Focus
(c) Radius of curvature
(d) Principal axis
A light ray falling at an angle of 45° with the surface of a clean slab of ice of thickness 1.00 m is refracted into it at an angle of 30°. Calculate the time taken by the light rays to cross the slab. Speed of light in vacuum = 3 × 108 m s−1.
A small object is placed at the centre of the bottom of a cylindrical vessel of radius 3 cm and height 4 cm filled completely with water. Consider the ray leaving the vessel through a corner. Suppose this ray and the ray along the axis of the vessel are used to trace the image. Find the apparent depth of the image and the ratio of real depth to the apparent depth under the assumptions taken. Refractive index of water = 1.33.
A light ray is incident normally on the face AB of a right-angled prism ABC (μ = 1.50) as shown in figure. What is the largest angle ϕ for which the light ray is totally reflected at the surface AC?

A light ray, going through a prism with the angle of prism 60°, is found to deviate by 30°. What limit on the refractive index can be put from these data?
The focal length f is related to the radius of curvature r of the spherical convex mirror by ______.
Car B overtakes car A at a relative speed of 40 ms-1. How fast will the image of car B appear to move in the mirror of focal length 10 cm fitted in car A, when car B is 1.9 m away from car A?
A point object is placed at a distance of 30 cm from a convex mirror of a focal length of 30 cm. What is the separation between the image and the object?
A convex lens of focal length 15 cm is placed coaxially in front of a convex mirror. The lens is 5 cm from the pole of the mirror. When an object is placed on the axis at a distance of 20 cm from the lens, it is found that the image coincides with the object. Calculate the radius of curvature of the mirror - (consider all-optical event):
