Advertisements
Advertisements
प्रश्न
k transparent slabs are arranged one over another. The refractive indices of the slabs are μ1, μ2, μ3, ... μk and the thicknesses are t1 t2, t3, ... tk. An object is seen through this combination with nearly perpendicular light. Find the equivalent refractive index of the system which will allow the image to be formed at the same place.
उत्तर
k number of transparent slabs are arranged one over the other.
Refractive indices of the slabs = μ1, μ2, μ3, ..., μk
Thickness of the slabs = t1, t2, t3,.., tk
Shift due to one slab: \[∆ t = \left[ 1 - \frac{1}{\mu} \right]t\]
For the combination of multiple slabs, the shift is given by,
\[∆ t = \left[ 1 - \frac{1}{\mu_1} \right] t_1 + \left[ 1 - \frac{1}{\mu_2} \right] t_2 + . . . + \left[ 1 - \frac{1}{\mu_k} \right] t_k . . . (i)\]|
Let μ be the refractive index of the combination of slabs.
The image is formed at the same place.
So, the shift will be:
\[\Delta t = \left[ 1 - \left( \frac{1}{\mu} \right) \right]( t_1 + t_2 . . . + t_k ) . . . (ii)\]
Equating (i) and (ii), we get:
\[\left[ 1 - \left( \frac{1}{\mu} \right) \right]( t_1 + t_2 . . . + t_k ) = \left[ 1 - \frac{1}{\mu_1} \right] t_1 + \left[ 1 - \frac{1}{\mu_2} \right] t_2 + \left[ 1 - \frac{1}{\mu_k} \right] t_k \]
\[ = ( t_1 + t_2 . . . + t_k ) - \left( \frac{t_1}{\mu_1} + \frac{t_2}{\mu_2} + \frac{t_k}{\mu_k} \right)\]
\[ = - \frac{1}{\mu} \sum^k_{i = 1} t_i = - \sum^k_{i = 1} \left( \frac{t_i}{\mu_i} \right)\]
\[ \Rightarrow \mu = \frac{\sum^k_{i = 1} t_i}{- \sum^k_{i = 1} \left( \frac{t_i}{\mu_i} \right)}\]
Hence, the required equivalent refractive index is
\[\frac{\sum^k_{i = 1} t_i}{\sum^k_{i = 1} \left( \frac{t_i}{\mu_i} \right)}\]
APPEARS IN
संबंधित प्रश्न
Draw the intensity distribution for the diffraction bands produced due to single slit ?
In the meterbridge experimental set up, shown in the figure, the null point ‘D’ is obtained at a distance of 40 cm from end A of the meterbridge wire. If a resistance of 10Ω is connected in series with R1, null point is obtained at AD = 60 cm. Calculate the values of R1 and R2.
Suppose you are inside the water in a swimming pool near an edge. A friends is standing on the edge. Do you find your friend taller or shorter than his usual height?
The image formed by a concave mirror
A thin lens is made with a material having refractive index
\[\mu = 1 \cdot 5\]. Both the side are convex. It is dipped in water \[\mu = 1 \cdot 33\]. It will behave like
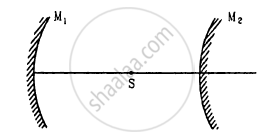
A converging mirror M1, a point source S and a diverging mirror M2 are arranged as shown in figure. The source is placed at a distance of 30 cm from M1. The focal length of each of the mirrors is 20 cm. Consider only the images formed by a maximum of two reflections. It is found that one image is formed on the source itself. (a) Find the distance between the two mirrors. (b) Find the location of the image formed by the single reflection from M2.
Light falls from glass (μ = 1.5) to air. Find the angle of incidence for which the angle of deviation is 90°.
A point source is placed at a depth h below the surface of water (refractive index = μ). (a) Show that light escapes through a circular area on the water surface with its centre directly above the point source. (b) Find the angle subtended by a radius of the area on the source.
A biconvex thick lens is constructed with glass (μ = 1.50). Each of the surfaces has a radius of 10 cm and the thickness at the middle is 5 cm. Locate the image of an object placed far away from the lens.
The diameter of the sun is 1.4 × 109 m and its distance from the earth is 1.5 × 1011 m. Find the radius of the image of the sun formed by a lens of focal length 20 cm.
Explain: ‘How is a rainbow formed’?
Answer the following question in detail.
State the conditions under which a rainbow can be seen.
Answer the following question in detail.
Explain the formation of a secondary rainbow. For which angular range with the horizontal is it visible?
Rainbow is the phenomenon due to ______.
Explain the formation of primary and secondary rainbow.
| Case study: Mirage in deserts |
 |
|
To a distant observer, the light appears to be coming from somewhere below the ground. The observer naturally assumes that light is being reflected from the ground, say, by a pool of water near the tall object. Such inverted images of distant tall objects cause an optical illusion to the observer. This phenomenon is called mirage. This type of mirage is especially common in hot deserts. Based on the above facts, answer the following question: |
A diamond is immersed in such a liquid which has its refractive index with respect to air as greater than the refractive index of water with respect to air. Then the critical angle of diamond-liquid interface as compared to critical angle of diamond-water interface will
| Case study: Mirage in deserts |
 |
|
To a distant observer, the light appears to be coming from somewhere below the ground. The observer naturally assumes that light is being reflected from the ground, say, by a pool of water near the tall object. Such inverted images of distant tall objects cause an optical illusion to the observer. This phenomenon is called mirage. This type of mirage is especially common in hot deserts. Based on the above facts, answer the following question: |
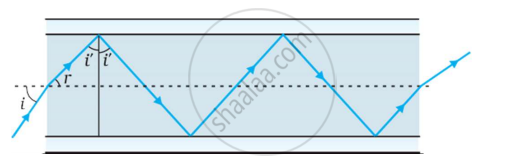
The following figure shows a cross-section of a ‘light pipe’ made of a glass fiber of refractive index 1.68. The outer covering of the pipe is made of a material of refractive index 1.44. What is the range of the angles of the incident rays with the axis of the pipe for the following phenomena to occur.
The sky would appear red instead of blue if
A short pulse of white light is incident from air to a glass slab at normal incidence. After travelling through the slab, the first colour to emerge is ______.
