Advertisements
Advertisements
प्रश्न
A line 5x + 3y + 15 = 0 meets y-axis at point P. Find the co-ordinates of points P. Find the equation of a line through P and perpendicular to x – 3y + 4 = 0.
उत्तर
P lies on y-axis and let the co-ordinates of P be (0, y)
∵ P lies also on the line 5x + 3y + 15 = 0
∴ It will satisfy it.
∴ 5 × 0 + 3y + 15 = 0 `\implies` 3y = –15
∴ y = –5
∴ Co-ordinates of P are (0, –5)
Now, writing the line x – 3y + 4 = 0 is form of y = mx + c
–3y = –x – 4
`\implies` 3y = x + 4
`\implies y = 1/3 x + 4/3`
∴ Slope of given line = `1/3`
∴ Slope of the line which is perpendicular to it
= `-(3/1)`
= –3 ...(∵ Product of slopes = –1)
∴ Equation of the line passing through P(0, –5)
y – y1 = m(x – x1)
`\implies` y + 5 = –3(x – 0)
`\implies` y + 5 = –3x
`\implies` 3x + y + 5 = 0
APPEARS IN
संबंधित प्रश्न
State, true or false :
The point (–3, 0) lies on the line x + 3 = 0
The co-ordinates of two points A and B are (–3, 4) and (2, –1). Find:
- the equation of AB;
- the co-ordinates of the point where the line AB intersects the y-axis.
A(1, 4), B(3, 2) and C(7, 5) are vertices of a triangle ABC. Find the co-ordinates of the centroid of triangle ABC.
A(1, 4), B(3, 2) and C(7, 5) are vertices of a triangle ABC. Find the equation of a line, through the centroid and parallel to AB.
The line 7x - 8y = 4 divides join of (-8,-4) and (6,k) in the ratio of 2 : 5. Find the value of k.
L is a point on the line segment PQ dividing it in the ratio 1 : 3. If the coordinates of P and Q are (3, 7) and ( 11,-5) respectively, find if L lies on the line 2x + 5y = 20.
Find the value of a line parallel to the following line:
x = `"y"/2` - 5
Find the equation of a line whose slope and y-intercept are m = -3, c = -1
Find the equation of the line passing through (0, 4) and parallel to the line 3x + 5y + 15 = 0.
In the adjoining figure, write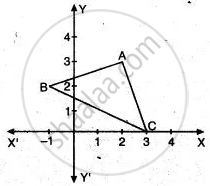
(i) The coordinates of A, B and C.
(ii) The equation of the line through A and | | to BC.
