Advertisements
Advertisements
प्रश्न
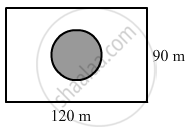
A park is in the form of a rectangle 120 m by 90 m. At the centre of the park there is a circular lawn as shown in the figure. The area of the park excluding the lawn is 2950 m2. Find the radius of the circular lawn.
उत्तर
Area of the rectangle = l × b
= 120 × 90
= 10800 sq. m
Area of the park excluding the lawn = 2950 m2
Area of the circular lawn = Area of the park -- Area of the park excluding the lawn
= 10800 - 2950
= 7850 m2
Area of the circular lawn =πr2
`=> 7850 = 22/7xx"r"^2`
`=> (7850) = 22/7xx"r"^2`
`=> "r"^2 = 2497.72`
⇒ r = 49.97
Or,
r ≈ 50 m
Thus, the radius of the circular lawn is 50 m.
APPEARS IN
संबंधित प्रश्न
A chord of a circle of radius 15 cm subtends an angle of 60° at the centre. Find the areas of the corresponding minor and major segments of the circle. [Use π = 3.14 and `sqrt3 = 1.73`]
A sector of a circle of radius 8cm contains the angle of 135°. Find the area of sector.
In a circle of radius 35 cm, an arc subtends an angle of 72° at the centre. Find the length of arc and area of sector
In the given figure, three sectors of a circle of radius 7 cm, making angles of 60°, 80° and 40° at the centre are shaded. Find the area of the shaded region.

In the given figure, APB and CQD are semicircles of diameter 7 cm each, while ARC an BSD are semicircles of diameter 14 cm each. Find the
- perimeter
- area of the shaded region.
Four cows are tethered at the four corners of a square field of side 50 m such that the each can graze the maximum unshared area. What area will be left ungrazed?
If the area of a circle is numerically equal to twice its circumference, then the diameter of the circle is ____________.
The area of the shaded portion in the following figure is equal to the area of.
In figure, a square is inscribed in a circle of diameter d and another square is circumscribing the circle. Is the area of the outer square four times the area of the inner square? Give reasons for your answer.
What is the length of arc of a circle of radius 7 cm which subtends an angle of 90° at the centre of the circle?
