Advertisements
Advertisements
प्रश्न
A rod of length L is pivoted at one end and is rotated with a uniform angular velocity in a horizontal plane. Let T1 and T2 be the tensions at the points L/4 and 3L/4 away from the pivoted ends.
विकल्प
T1 > T2
T2 > T1
T1 = T2
The relation between T1 and T2 depends on whether the rod rotates clockwise or anticlockwise.
उत्तर
T1 > T2
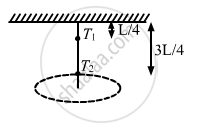
Let the angular velocity of the rod be \[\omega\] .
Distance of the centre of mass of portion of the rod on the right side of L/4 from the pivoted end :
\[r_1 = \frac{L}{4} + \frac{1}{2}\left( \frac{3L}{4} \right) = \frac{5L}{8}\]
Mass of the rod on the right side of L/4 from the pivoted end : \[\text{m}_1 = \frac{3}{4}\text{M}\]
At point L/4, we have :
\[T_1 = \text{ m}_1 \omega^2 \text{ r}_1 \]
\[ = \frac{3}{4}\text{ M } \omega^2 \frac{5}{8}\text{ L} = \frac{15}{32}\text{ M }\omega^2 \text{ L}\]
Distance of the centre of mass of rod on the right side of 3L/4 from the pivoted end :
\[\text{r}_1 = \frac{1}{2}\left( \frac{L}{4} \right) + \frac{3L}{4} = \frac{7L}{8}\]
Mass of the rod on the right side of L/4 from the pivoted end : \[\text{m}_1 = \frac{1}{4}\text{M}\]
At point 3L/4, we have :
\[\text{T}_2 = \text{m}_2 \omega^2 \text{r}_2 \]
\[ = \frac{1}{4}\text{M} \omega^2 \frac{7}{8}\text{L} = \frac{7}{32}\text{M} \omega^2 \text{L}\]
∴ T1 > T2
APPEARS IN
संबंधित प्रश्न
A stone of mass 0.25 kg tied to the end of a string is whirled round in a circle of radius 1.5 m with a speed of 40 rev/min in a horizontal plane. What is the tension in the string? What is the maximum speed with which the stone can be whirled around if the string can withstand a maximum tension of 200 N?
A disc revolves with a speed of `33 1/3` rev/min, and has a radius of 15 cm. Two coins are placed at 4 cm and 14 cm away from the centre of the record. If the co-efficient of friction between the coins and the record is 0.15, which of the coins will revolve with the record?
A particle is kept fixed on a turntable rotating uniformly. As seen from the ground the particle goes in a circle, its speed is 20 cm/s and acceleration is 20 cm/s2. The particle is now shifted to a new position to make the radius half of the original value. The new value of the speed and acceleration will be
A coin placed on a rotating turntable just slips. If it is placed at a distance of 4 cm from the centre. If the angular velocity of the turntable is doubled, it will just slip at a distance of
Three identical cars A, B and C are moving at the same speed on three bridges. The car A goes on a place bridge, B on a bridge convex upward and C goes on a bridge concave upward. Let FA, FB and FC be the normal forces exerted by the car on the bridges when they are at the middle of bridges.
Assume that the earth goes round the sun in a circular orbit with a constant speed of 30 kms
A particle is going in a spiral path as shown in figure with constant speed.
A person stands on a spring balance at the equator. By what fraction is the balance reading less than his true weight?
A turn of radius 20 m is banked for the vehicles going at a speed of 36 km/h. If the coefficient of static friction between the road and the tyre is 0.4, what are the possible speeds of a vehicle so that it neither slips down nor skids up?
A hemispherical bowl of radius R is rotated about its axis of symmetry which is kept vertical. A small block is kept in the bowl at a position where the radius makes an angle θ with the vertical. The block rotates with the bowl without any slipping. The friction coefficient between the block and the bowl surface is μ. Find the range of the angular speed for which the block will not slip.
A particle is projected with a speed u at an angle θ with the horizontal. Consider a small part of its path near the highest position and take it approximately to be a circular arc. What is the radius of this circular circle? This radius is called the radius of curvature of the curve at the point.
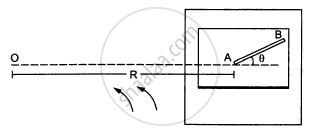
A table with smooth horizontal surface is fixed in a cabin that rotates with a uniform angular velocity ω in a circular path of radius R (In the following figure). A smooth groove AB of length L(<<R) is made the surface of the table. The groove makes an angle θ with the radius OA of the circle in which the cabin rotates. A small particle is kept at the point A in the groove and is released to move at the point A in the groove and is released to move along AB. Find the time taken by the particle to reach the point B.
A body slides down a smooth inclined plane having angle θ and reaches the bottom with velocity v. If a body is a sphere, then its linear velocity at the bottom of the plane is
A rigid body is rotating with angular velocity 'ω' about an axis of rotation. Let 'v' be the linear velocity of particle which is at perpendicular distance 'r' from the axis of rotation. Then the relation 'v = rω' implies that ______.
A body of mass m is performing a UCM in a circle of radius r with speed v. The work done by the centripetal force in moving it through `(2/3)`rd of the circular path is ______.
A body of M.I. 2 kg m2 rotates with an angular velocity of 20 rad/s. When an external torque of 0.5 N m acts on it in the opposite direction, the number of revolutions it makes before it comes to rest is ____________.
A body is moving along a circular track of radius 100 m with velocity 20 m/s. Its tangential acceleration is 3 m/s2 then its resultant accelaration will be ______.
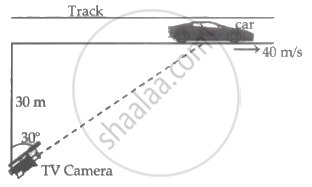
A racing car is travelling along a track at a constant speed of 40 m/s. A T.V. cameraman is recording the event from a distance of 30 m directly away from the track as shown in the figure. In order to keep the car under view in the position shown, the angular speed with which the camera should be rotated is ______.
Statement I: A cyclist is moving on an unbanked road with a speed of 7 kmh-1 and takes a sharp circular turn along a path of radius of 2 m without reducing the speed. The static friction coefficient is 0.2. The cyclist will not slip and pass the curve. (g = 9.8 m/s2)
Statement II: If the road is banked at an angle of 45°, cyclist can cross the curve of 2 m radius with the speed of 18.5 kmh-1 without slipping.
In the light of the above statements, choose the correct answer from the options given below.
