Advertisements
Advertisements
प्रश्न
A short object of length L is placed along the principal axis of a concave mirror away from focus. The object distance is u. If the mirror has a focal length f, what will be the length of the image? You may take L << |v – f|.
Advertisements
उत्तर
As the mean distance of object from mirror is u
∴ `u_1 = u - L/2` and `u_2 = (u + L/2)`
Let the image of the two ends of object form at distance v1 and v2(v1 > v2). So length of image on principal axis is L' = (v1 – v2)
`1/v + 1/u = 1/f` or `1/v + 1/f = 1/u`
⇒ `1/v = (u - f)/(uf)`
⇒ `v = (uf)/(u - f)`
So L' = `v_1 - v_2 = ((u - L/2)f)/((u - L/2) - f) - ((u + L/2)f)/((u + L/2) - f)`
⇒ L' = `f (u - L/2)/((u - f - L/2)) - (u + 1/2)/((u - f + L/2))`
⇒ L' = `f[((u - L/2)(u - f + L/2) - (u + L/2)(u - f - L/2))/((u - f - L/2)(u - f + 1/2))]`
= `(f[u^2 - uf + (uL)/2 - (uL)/2 + (fL)/2 - L^2/4 - (u^2 - uf - (uL)/2 + (uL)/2 - (fL)/2 - L^2/4)])/((u - f)^2 - L^2/4)`
∴ `L < < (u - f)`
∴ `L^2/4 < < < (u - f)`
So neglecting the terms `L^2/4`
`L^2 = f[((fL)/2 + (fL)/2)/(u - f)^2]`
`L^2 = (ffL)/((u - f)^2` ⇒ `L^2 = (Lf^2)/((u - f)^2`
It is the length of image f.
APPEARS IN
संबंधित प्रश्न
A 4.5 cm needle is placed 12 cm away from a convex mirror of focal length 15 cm. Give the location of the image and the magnification. Describe what happens as the needle is moved farther from the mirror.
If an object far away from a convex mirror moves towards the mirror, the image also moves. Does it move faster, slower or at the same speed as compared to the object?
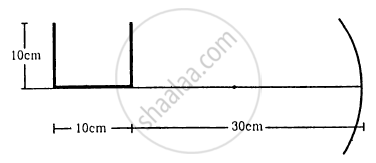
A U-shaped wire is placed before a concave mirror having radius of curvature 20 cm as shown in figure. Find the total length of the image.
A diverging lens of focal length 20 cm and a converging mirror of focal length 10 cm are placed coaxially at a separation of 5 cm. Where should an object be placed so that a real image is formed at the object itself?
A converging lens of focal length 40 cm is kept in contact with a diverging lens of focal length 30 cm. Find the focal length of the combination .
How can the spherical aberration produced by a lens be minimized?
Two thin lenses having optical powers of -10D and+ 6D are placed in contact with each other. The focal length of the combination is:
State how the focal length of a glass lens (Refractive Index 1.5) changes when it is completely immersed in:
(i) Water (Refractive Index 1.33)
(ii) A liquid (Refractive Index 1.65)
According to the mirror equation, ______.
The radius of curvature of the curved surface of a plano-convex lens is 20 cm. If the refractive index of the material of the lens be 1.5, it will ______.
An astronomical refractive telescope has an objective of focal length 20 m and an eyepiece of focal length 2 cm.
- The length of the telescope tube is 20.02 m.
- The magnification is 1000.
- The image formed is inverted.
- An objective of a larger aperture will increase the brightness and reduce chromatic aberration of the image.
A thin convex lens of focal length 25 cm is cut into two pieces 0.5 cm above the principal axis. The top part is placed at (0, 0) and an object placed at (– 50 cm, 0). Find the coordinates of the image.
A spherical mirror is obtained as shown in the figure from a hollow glass sphere. if an object is positioned in front of the mirror, what will be the nature and magnification of the image of the object? (Figure drawn as schematic and not to scale)
A concave mirror of focal length 12 cm forms three times the magnified virtual image of an object. Find the distance of the object from the mirror.
For a spherical mirror, the normal at the point of incidence is:
The angle of incidence is the angle between:
The angle of reflection is the angle between:
According to the laws of reflection, the incident ray, the reflected ray, and the normal to the reflecting surface at the point of incidence:
The laws of reflection are valid:
