Advertisements
Advertisements
प्रश्न
A solid is in the shape of a cone standing on a hemisphere with both their radii being equal to 7 cm and the height of the cone is equal to its diameter. Find the volume of the solid. [Use`pi22/7`]
उत्तर
Let r and h be radius and height of the cone respectively.
Radius of cone (r) = 7 cm (Given)
Diameter of cone = 2 × r = (2 × 7) cm = 14 cm
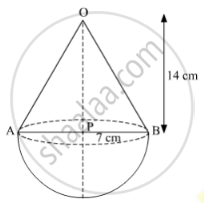
According to the question, height of the cone is equal to its diameter.
∴ Height of cone (h) = 14 cm
Radius of hemisphere = Radius of cone = 7 cm
∴ Volume of solid = Volume of cone + Volume of hemisphere
`=1/3pir^2h+2/3pir^3`
`=(pir^2)/3[h+2r]`
`=1/3xx22/7xx7xx7xx[14+(2xx7)]cm^2`
`=22/3xx7xx28cm^3`
`=4312/2cm^2`
`=1437.33cm^3`
Thus, the volume of the solid is 1437.33 cm3.
APPEARS IN
संबंधित प्रश्न
Metal spheres each of radius 2cm are packed into a rectangular box of internal dimension 16cm x 8cm x 8cm when 16 spheres are packed the box is filled with preservative liquid. Find volume of this liquid?
The surface area of a solid metallic sphere is 616 cm2. It is melted and recast into a cone of height 28 cm. Find the diameter of the base of the cone so formed (Use it =`22/7`)
12 spheres of the same size are made from melting a solid cylinder of 16 cm diameter and 2 cm height. The diameter of each sphere is
A cylindrical container of radius 6 cm and height 15 cm is filled with ice-cream. The whole ice-cream has to be distributed to 10 children in equal cones with hemispherical tops. If the height of the conical portion is 4 times the radius of its base, then find the radius of the ice-cream cone.
The radii of internal and external surfaces of a hollow spherical shell are 3 cm and 5 cm, respectively. It is melted and recast into a solid cylinder of diameter 14 cm. Find the height of the cylinder.
A wooden toy was made by scooping out a hemisphere of same radius from each end of a solid cylinder. If the height of the cylinder is 10 cm and its base is of radius 3.5 cm, then find the volume of wood in the toy.
A surahi is a combination of
The diameter of a sphere is 14 cm. Its volume is
How many spherical lead shots each of diameter 4.2 cm can be obtained from a solid rectangular lead piece with dimensions 66 cm, 42 cm and 21 cm.
A solid iron cuboidal block of dimensions 4.4 m × 2.6 m × 1 m is recast into a hollow cylindrical pipe of internal radius 30 cm and thickness 5 cm. Find the length of the pipe.
