Advertisements
Advertisements
प्रश्न
A straight line is drawn cutting two equal circles and passing through the mid-point M of the line joining their centers O and O'. Prove that the chords AB and CD, which are intercepted by the two circles, are equal.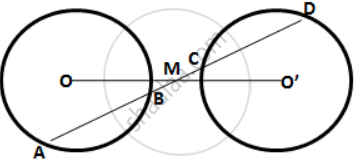
उत्तर
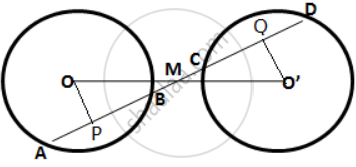
Given: A straight line AD intersects two circles of equal radii at A, B, C and D.
The line joining the centers OO' intersect AD at M and M is the midpoint of OO'.
To Prove: AB = CD.
Construction: From O, draw OP ⊥ AB and from O', draw O'Q ⊥ CD.
Proof:
In ΔOMP and ΔO'MQ,
∠OMP = ∠O'MQ ...( Vertically Opposite angles )
∠OPM = ∠O'QM ...( each = 90° )
OM = O'M ...( Given )
By Angle-Angle-Side criterion of congruence,
∴ ΔOMP ≅ ΔO'MQ, ...( by AAS )
The corresponding parts of the congruent triangles are congruent.
∴ OP = O'Q ...( c.p.c.t. )
We know that two chords of a circle or equal circles which are equidistant from the center are equal.
∴ AB = CD.
APPEARS IN
संबंधित प्रश्न
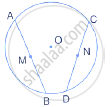
M and N are the mid-points of two equal chords AB and CD respectively of a circle with centre O. prove that:
(i) ∠BMN = ∠DNM.
(ii) ∠AMN = ∠CNM.
OABC is a rhombus whose three vertices A, B and C lie on a circle with centre O. If the area of the rhombus is `32sqrt(3) cm^2` find the radius of the circle.
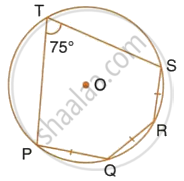
The given figure shows a circle with centre O. Also, PQ = QR = RS and ∠PTS = 75°.
Calculate:
- ∠POS,
- ∠QOR,
- ∠PQR.
In fig. the centre of the circle is O. PQ and RS are two equal chords of the circle which , when produced , meet at T outside the circle . Prove that (a) TP = TR (b) TQ = TS.

Two congruent drdes have their centres at 0 and P. Mis the midpoint of the line segment OP. A straight line is drawn through M cutting the two circles at the points A, B, C and D. Prove that the chords AB and CD are equal.
In fig, AB and CD are two equal chords of a circle with centre O. If M and N are the midpoints of AB and CD respectively,
prove that (a) ∠ ONM = ∠ ONM (b) ∠ AMN = ∠ CNM.
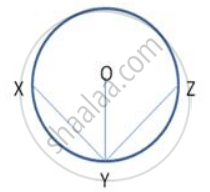
In following figure .,XY and YZ are two equal chords of a circle with centre O. Prove that the bisector of ∠ XYZ passes through O.
AB and AC are two equal chords of a circle with centre o such that LABO and LCBO are equal. Prove that AB = BC.
Two equal chords AB and CD of a circle with center O, intersect each other at point P inside the circle.
Prove that: (i) AP = CP ; (ii) BP = DP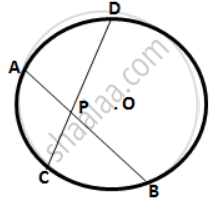
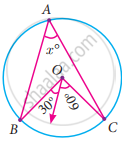
Find the value of x° in the following figure: