Advertisements
Advertisements
प्रश्न
A tent of height 77dm is in the form a right circular cylinder of diameter 36m and height 44dm surmounted by a right circular cone. Find the cost of canvas at Rs.3.50 per m2 ?
उत्तर
Given that height of a tent = 77dm
Height of cone = 44dm
Height of a tent without cone = 77 - 44 = 33dm
= 3.3m
Given diameter of cylinder (d) = 36m
Radius (r) = `36/2`= 18m
Let ‘l’ be slant height of cone
`l^2=r^2+h^2`
`l^2=18^2+3.3^2`
l2 = 324 + 10.89
l2 = 334.89
l = 18.3
Slant height of cone l = 18.3
Curved surface area of cylinder (S1) = 2πrh
= 2 x π x18 x 4.4m2 ............(1)
Curved surface area of cone (S2) = πrl
= π18 x 18.3m2 .............(2)
Total curved surface of tent = S1 + S2
T.C.S.A = S1 + S2
= 1532.46m2
Given cost canvas per m2 = RS 3.50
Total cost of canvas per 1532.46 X 3.50
= 1532.46 X 3.50
= 5363.61
∴ Total cost of canvas = Rs 5363.61
संबंधित प्रश्न
In Fig. 4, from the top of a solid cone of height 12 cm and base radius 6 cm, a cone of height 4 cm is removed by a plane parallel to the base. Find the total surface area of the remaining solid. (Use `pi=22/7` and `sqrt5=2.236`)

Radii of circular ends of a solid frustum off a cone re 33cm and 27cm and its slant height are 10cm. find its total surface area?
In Figure 4, from a rectangular region ABCD with AB = 20 cm, a right triangle AED with AE = 9 cm and DE = 12 cm, is cut off. On the other end, taking BC as diameter, a semicircle is added on outside the region. Find the area of the shaded region.\[[Use\pi = 3 . 14]\]

If r1 and r2 be the radii of two solid metallic spheres and if they are melted into one solid sphere, prove that the radius of the new sphere is \[\left( r_1^3 + r_2^3 \right)^\frac{1}{3}\].
A hemispherical bowl of internal diameter 30 cm contains some liquid. This liquid is to be poured into cylindrical bottles of diameter 5 cm and height 6 cm each. Find the number of bottles required.
A spherical ball of radius 3 cm is melted and recast into three spherical balls. The radii of two of these balls are 1.5 cm and 2 cm. Find the radius of the third ball.
Water is flowing through a cylindrical pipe of internal diameter 2 cm, into a cylindrical tank of base radius 40 cm, at the rate of 0.4 m per second. Determine the rise in level of water in the tank in half an hour.
The volume of a hemisphere is 19404 cm3. The total surface area of the hemisphere is
Two cubes each of volume 8 cm³ are joined end to end, then the surface area of the resulting cuboid is ______.
|
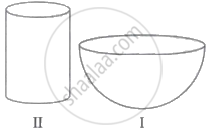
Khurja is a city in the Indian state of Uttar Pradesh famous for the pottery. Khurja pottery is traditional Indian pottery work which has attracted Indians as well as foreigners with a variety of tea sets, crockery and ceramic tile works. A huge portion of the ceramics used in the country is supplied by Khurja and is also referred as "The Ceramic Town". One of the private schools of Bulandshahr organised an Educational Tour for class 10 students to Khurja. Students were very excited about the trip. Following are the few pottery objects of Khurja.
Students found the shapes of the objects very interesting and they could easily relate them with mathematical shapes viz sphere, hemisphere, cylinder etc. |
Maths teacher who was accompanying the students asked the following questions:
- The internal radius of hemispherical bowl (filled completely with water) in I is 9 cm and the radius and height of the cylindrical jar in II are 1.5 cm and 4 cm respectively. If the hemispherical bowl is to be emptied in cylindrical jars, then how many cylindrical jars are required?
- If in the cylindrical jar full of water, a conical funnel of the same height and same diameter is immersed, then how much water will flow out of the jar?