Advertisements
Advertisements
प्रश्न
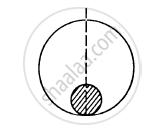
A uniform metal sphere of radius a and mass M is surrounded by a thin uniform spherical shell of equal mass and radius 4a (In the following figure). The centre of the shell falls on the surface of the inner sphere. Find the gravitational field at the points P1 and P2 shown in the figure.
Advertisements
उत्तर
At point P1, the gravitational field due to the sphere and the shell is given by
F \[= \frac{GM}{\left( 3a + a \right)^2} + 0 = \frac{GM}{16 a^2}\]
At point P2, the gravitational field due to the sphere and the shell is given by
\[F = \frac{GM}{\left( a + 4a + a \right)^2} + \frac{GM}{\left( 4a + a \right)^2}\]
\[ \Rightarrow F = \frac{GM}{36 a^2} + \frac{GM}{25 a^2}\]
\[ \Rightarrow F = \frac{GM}{a^2}\left( \frac{1}{36} + \frac{1}{25} \right)\]
\[ \Rightarrow F = \frac{GM}{a^2}\left( \frac{25 + 36}{900} \right)\]
\[ \Rightarrow F = \left( \frac{61}{900} \right)\frac{GM}{a^2}\]
APPEARS IN
संबंधित प्रश्न
Write the answer of the question with reference to laws of gravitation.
State the universal law of gravitation.
Write the formula to find the magnitude of the gravitational force between the earth and an object on the surface of the earth.
Calculate the force of gravitation between the earth and the Sun, given that the mass of the earth = 6 × 1024 kg and of the Sun = 2 × 1030 kg. The average distance between the two is 1.5 × 1011 m.
If you compare the gravitational force on the Earth due to the Sun to that due to the Moon, you would find that the Sun’s pull is greater than the Moon’s pull. (You can check this yourself using the data available in the succeeding exercises). However, the tidal effect of the Moon’s pull is greater than the tidal effect of Sun. Why?
Three uniform spheres each having a mass M and radius a are kept in such a way that each touches the other two. Find the magnitude of the gravitational force on any of the spheres due to the other two.
Two small bodies of masses 10 kg and 20 kg are kept a distance 1.0 m apart and released. Assuming that only mutual gravitational forces are acting, find the speeds of the particles when the separation decreases to 0.5 m.
The gravitational field in a region is given by \[E = \left( 2 \overrightarrow{i} + 3 \overrightarrow{j} \right) N {kg}^{- 1}\] . Show that no work is done by the gravitational field when a particle is moved on the line 3y + 2x = 5.
[Hint : If a line y = mx + c makes angle θ with the X-axis, m = tan θ.]
The law of gravitation gives the gravitational force between :
Where will you weigh more: at the centre of the earth or at the surface of the earth?
Name and state the action and reaction in the following case:
A book lying on a table.
Why does a ball moving on a table top eventually stops?
Explain why:
The atmosphere does not escape.
Three uniform spheres, each having mass m and radius r, are kept in such a way that each touches the other two. The magnitude of the gravitational force on any sphere due to the other two is
Particles of masses 2M, m and M are respectively at points A, B and C with AB = ½ (BC). m is much-much smaller than M and at time t = 0, they are all at rest (Figure). At subsequent times before any collision takes place ______.
If the law of gravitation, instead of being inverse-square law, becomes an inverse-cube law- ______.
- planets will not have elliptic orbits.
- circular orbits of planets is not possible.
- projectile motion of a stone thrown by hand on the surface of the earth will be approximately parabolic.
- there will be no gravitational force inside a spherical shell of uniform density.
The gravitational force between a hollow spherical shell (of radius R and uniform density) and a point mass is F. Show the nature of F vs r graph where r is the distance of the point from the centre of the hollow spherical shell of uniform density.
Six point masses of mass m each are at the vertices of a regular hexagon of side l. Calculate the force on any of the masses.
Write the answer of the question with reference to laws of gravitation.
Write the value of the universal gravitational constant.
Two particles of equal mass 'm' go around a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle with respect to its centre of mass is ______.
If the distance between two objects is doubled, the gravitational force becomes ______.
