Question

A solid sphere of mass m and radius r is placed inside a hollow thin spherical shell of mass M and radius R as shown in the following figure . A particle of mass m' is placed on the line joining the two centres at a distance x from the point of contact of the sphere and the shell. Find the magnitude of the resultant gravitational force on this particle due to the sphere and the shell if (a) r < x < 2r, (b) 2r < x < 2R and (c) x > 2R.

Answer 1

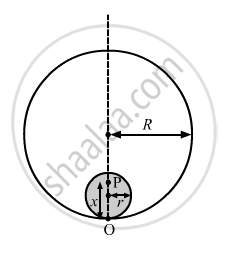
(a) Consider that the particle is placed at a distance x from O.

Here, r < x < 2r
Let us consider a thin solid sphere of radius (x \[-\] r).

Mass of the sphere,

\[dm = \frac{m}{\left( \frac{4}{3} \right)\pi r^3} \times \frac{4}{3}\pi(x - r )^3 = \frac{m(x - r )^3}{r^3}\]

Then the gravitational force on the particle due to the solid sphere is given by

\[F = \frac{Gm' dm}{(x - r )^2}\]

\[ = \frac{G\frac{m(x - r )^3}{r^3}m'}{(x - r )^2} = \frac{Gmm'(x - r)}{r^3}\]

Force on the particle due to the shell will be zero because gravitational field intensity inside a shell is zero.

(b)  If 2r < x < 2R,
Force on the body due to the shell will again be zero as particle is still inside the shell.
then F is only due to the solid sphere.

\[\therefore F = \frac{Gmm'}{\left( x - r \right)^2}\]

(c) If x > 2R, then the gravitational force is due to both the sphere and the shell.
Now, we have :
Gravitational force due to shell,

\[F = \frac{GMm'}{\left( x - R \right)^2}\]

Gravitational force due to the sphere \[= \frac{Gmm'}{\left( x - r \right)^2}\]

As both the forces are acting along the same line joining the particle with the centre of the sphere and shell so both the forces can be added directly without worrying about their vector nature.
∴ Resultant force \[= \frac{Gmm'}{\left( x - r \right)^2} + \frac{GMm'}{\left( x - R \right)^2}\]