Advertisements
Advertisements
प्रश्न
ΔABC is an isosceles triangle with AB = AC. D, E and F are the mid-points of BC, AB and AC respectively. Prove that the line segment AD is perpendicular to EF and is bisected by it.
उत्तर

Since the segment joining the mid-points of two sides of a triangle is parallel to third side and is half of it,
Therefore,
DE || AB, DE = `(1)/(2)"AB"`
Also,
DF || AC, DF = `(1)/(2)"AC"`
But AB = AC
⇒ `(1)/(2)"AB" = (1)/(2)"AC"`
⇒ DF = DE ........(i)
DE = `(1)/(2)"AB"`
⇒ DE = AF ........(ii)
And DF = `(1)/(2)"AC"`
⇒ DF = AE ........(iii)
From (i), (ii) and (iii)
DE = AE = EF = DF
⇒ DEAF is a rhombus.
⇒ Diagonals AD and EF bisect each other at right angles.
⇒ AD perpendicular to EF and AD is bisected by EF.
APPEARS IN
संबंधित प्रश्न
ABCD is a rhombus and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.
BM and CN are perpendiculars to a line passing through the vertex A of a triangle ABC. If
L is the mid-point of BC, prove that LM = LN.
In the given figure, `square`PQRS and `square`MNRL are rectangles. If point M is the midpoint of side PR then prove that,
- SL = LR
- LN = `1/2`SQ

In triangle ABC, M is mid-point of AB and a straight line through M and parallel to BC cuts AC in N. Find the lengths of AN and MN if Bc = 7 cm and Ac = 5 cm.
In ΔABC, D, E, F are the midpoints of BC, CA and AB respectively. Find ∠FDB if ∠ACB = 115°.
In a right-angled triangle ABC. ∠ABC = 90° and D is the midpoint of AC. Prove that BD = `(1)/(2)"AC"`.
In a parallelogram ABCD, M is the mid-point AC. X and Y are the points on AB and DC respectively such that AX = CY. Prove that:
(i) Triangle AXM is congruent to triangle CYM, and
(ii) XMY is a straight line.
ABCD is a parallelogram.E is the mid-point of CD and P is a point on AC such that PC = `(1)/(4)"AC"`. EP produced meets BC at F. Prove that: F is the mid-point of BC.
In the given figure, PS = 3RS. M is the midpoint of QR. If TR || MN || QP, then prove that: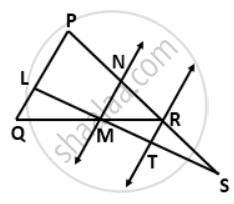
RT = `(1)/(3)"PQ"`
P, Q, R and S are respectively the mid-points of the sides AB, BC, CD and DA of a quadrilateral ABCD such that AC ⊥ BD. Prove that PQRS is a rectangle.
