Advertisements
Advertisements
प्रश्न
BM and CN are perpendiculars to a line passing through the vertex A of a triangle ABC. If
L is the mid-point of BC, prove that LM = LN.
उत्तर
To prove LM = LN
Draw LS perpendicular to line MN
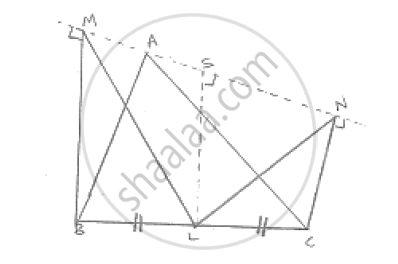
∴ The lines BM, LS and CN being the same perpendiculars, on line MN are parallel to each
other.
According to intercept theorem,
If there are three or more parallel lines and the intercepts made by them on a transversal or
equal. Then the corresponding intercepts on any other transversal are also equal.
In the drawn figure, MB and LS and NC are three parallel lines and the two transversal line
are MN and BC
We have, BL= LC (As L is the given midpoint of BC)
∴ using intercept theorem, we get
MS = SN ....(i )
Now in Δ MLS and LSN
MS = SN using ….(i)
`∠`LSM = `∠`LSN = 90°LS ^ MN and SL = LS common
∴ Δ DMLS ≅ Δ LSN (SAS congruency theorem)
∴ LM = LN (CPCT )
APPEARS IN
संबंधित प्रश्न
ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
ABCD is a square E, F, G and H are points on AB, BC, CD and DA respectively, such that AE = BF = CG = DH. Prove that EFGH is a square.
In a triangle, P, Q and R are the mid-points of sides BC, CA and AB respectively. If AC =
21 cm, BC = 29 cm and AB = 30 cm, find the perimeter of the quadrilateral ARPQ.
In Fig. below, BE ⊥ AC. AD is any line from A to BC intersecting BE in H. P, Q and R are
respectively the mid-points of AH, AB and BC. Prove that ∠PQR = 90°.

In parallelogram ABCD, P is the mid-point of DC. Q is a point on AC such that CQ = `(1)/(4)"AC"`. PQ produced meets BC at R. Prove that
(i) R is the mid-point of BC, and
(ii) PR = `(1)/(2)"DB"`.
Show that the quadrilateral formed by joining the mid-points of the adjacent sides of a square is also a square.
In the given figure, ABCD is a trapezium. P and Q are the midpoints of non-parallel side AD and BC respectively. Find: DC, if AB = 20 cm and PQ = 14 cm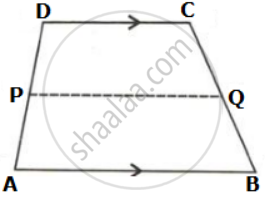
Side AC of a ABC is produced to point E so that CE = `(1)/(2)"AC"`. D is the mid-point of BC and ED produced meets AB at F. Lines through D and C are drawn parallel to AB which meets AC at point P and EF at point R respectively. Prove that: 4CR = AB.
In a parallelogram ABCD, E and F are the midpoints of the sides AB and CD respectively. The line segments AF and BF meet the line segments DE and CE at points G and H respectively Prove that: EGFH is a parallelogram.
E and F are respectively the mid-points of the non-parallel sides AD and BC of a trapezium ABCD. Prove that EF || AB and EF = `1/2` (AB + CD).
[Hint: Join BE and produce it to meet CD produced at G.]
