Advertisements
Advertisements
प्रश्न
ABCD is a parallelogram, E and F are the mid-points of AB and CD respectively. GH is any line intersecting AD, EF and BC at G, P and H respectively. Prove that GP = PH
उत्तर
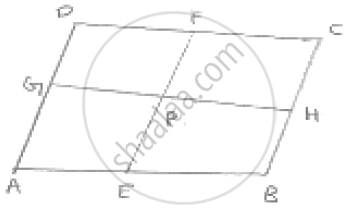
Since E and F are midpoints of AB and CD respectively
∴ AE = BE =`1/2` AB
And CF = DF =`1/2` CD
But, AB = CD
∴ `1/2` AB = `1/2` CD
⇒ BE = CF
Also, BE || CF [∵AB || CD]
∴ BEFC is a parallelogram
⇒ BC || EF and BF = PH ....(i )
Now, BC || EF
⇒ AD || EF [ ∵ BC || AD as ABCD is a parallel]
⇒ AEFD is parallelogram
⇒ AE = GP
But is the midpoint of AB
∴ AE = BE
⇒ GP = PH
APPEARS IN
संबंधित प्रश्न
In a ΔABC, E and F are the mid-points of AC and AB respectively. The altitude AP to BC
intersects FE at Q. Prove that AQ = QP.
ABC is a triang D is a point on AB such that AD = `1/4` AB and E is a point on AC such that AE = `1/4` AC. Prove that DE = `1/4` BC.
BM and CN are perpendiculars to a line passing through the vertex A of a triangle ABC. If
L is the mid-point of BC, prove that LM = LN.
In triangle ABC, M is mid-point of AB and a straight line through M and parallel to BC cuts AC in N. Find the lengths of AN and MN if Bc = 7 cm and Ac = 5 cm.
Prove that the figure obtained by joining the mid-points of the adjacent sides of a rectangle is a rhombus.
The diagonals AC and BD of a quadrilateral ABCD intersect at right angles. Prove that the quadrilateral formed by joining the midpoints of quadrilateral ABCD is a rectangle.
In ∆ABC, AB = 5 cm, BC = 8 cm and CA = 7 cm. If D and E are respectively the mid-points of AB and BC, determine the length of DE.
P, Q, R and S are respectively the mid-points of sides AB, BC, CD and DA of quadrilateral ABCD in which AC = BD and AC ⊥ BD. Prove that PQRS is a square.
Show that the quadrilateral formed by joining the mid-points of the consecutive sides of a square is also a square.
Prove that the line joining the mid-points of the diagonals of a trapezium is parallel to the parallel sides of the trapezium.
