Advertisements
Advertisements
प्रश्न
ABCD is a quadrilateral such that diagonal AC bisects the angles A and C. Prove that AB = AD and CB = CD.
उत्तर
Given: In a quadrilateral ABCD, diagonal AC bisects the angles A and C.
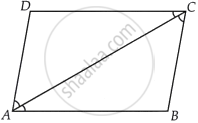
To prove: AB = AD and CB = CD
Proof: In ΔADC and ΔABC,
∠DAC = ∠BAC ...[∵ AC is the bisector of ∠A and ∠C]
∠DCA = ∠BCA ...[∵ AC is the bisector of ∠A and ∠C]
And AC = AC ...[Common side]
∴ ΔADC ≅ ΔABC ...[By ASA congruence rule]
AD = AB ...[By CPCT]
And CD = CB ...[By CPCT]
Hence proved.
APPEARS IN
संबंधित प्रश्न
ABC is a right angled triangle in which ∠A = 90° and AB = AC. Find ∠B and ∠C.
ΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see the given figure). If AD is extended to intersect BC at P, show that
- ΔABD ≅ ΔACD
- ΔABP ≅ ΔACP
- AP bisects ∠A as well as ∠D.
- AP is the perpendicular bisector of BC.

AD is an altitude of an isosceles triangles ABC in which AB = AC. Show that
- AD bisects BC
- AD bisects ∠A
Prove that in a quadrilateral the sum of all the sides is greater than the sum of its diagonals.
Prove that sum of any two sides of a triangle is greater than twice the median with respect to the third side.
In a right triangle, prove that the line-segment joining the mid-point of the hypotenuse to the opposite vertex is half the hypotenuse.
Two lines l and m intersect at the point O and P is a point on a line n passing through the point O such that P is equidistant from l and m. Prove that n is the bisector of the angle formed by l and m.
Line segment joining the mid-points M and N of parallel sides AB and DC, respectively of a trapezium ABCD is perpendicular to both the sides AB and DC. Prove that AD = BC.
ABC is a right triangle such that AB = AC and bisector of angle C intersects the side AB at D. Prove that AC + AD = BC.
ABCD is quadrilateral such that AB = AD and CB = CD. Prove that AC is the perpendicular bisector of BD.
