Advertisements
Advertisements
प्रश्न
An aeroplane when flying at a height of 3125 m from the ground passes vertically below another plane at an instant when the angles of elevation of the two planes from the same point on the ground are 30° and 60° respectively. Find the distance between the two planes at that instant.
उत्तर
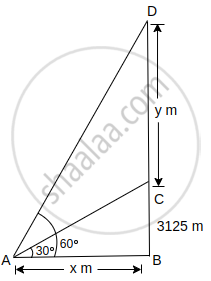
Let C and D be the two aeroplanes and A be the point of observation. Then,
∠CAB = 30°, ∠DAB = 60°, BC = 3125 m
Let DC = y m, AB = x m
In right ΔABC, ∠B = 90°
tan 30° = `("BC")/("AB")`
⇒ `1/sqrt(3) = 3125/("AB")`
⇒ AB = `3125sqrt(3)` m ...(i)
In right ΔABD, ∠B = 90°
tan 60° = `("BD")/("AB")`
⇒ `sqrt(3) = ("y"+ 3125)/(3125 sqrt(3))` ...[From equation (i)]
⇒ 3125 × 3 = y + 3125
⇒ y = 3125 (3 – 1)
⇒ y = 2 × 3125
⇒ y = 6250 m
Therefore, the distance between the two planes is 6250 m.
APPEARS IN
संबंधित प्रश्न
From a point on the ground 40 m away from the foot of a tower, the angle of elevation of the top of the tower is 30º. The angle of elevation of the top of a water tank (on the top of the tower) is 45º. Find the (i) height of the tower (ii) the depth of the tank.
A tree breaks due to storm and the broken part bends so that the top of the tree touches the ground, making an angle 30° with it. The distance between the foot of the tree to the point where the top touches the ground is 8 m. Find the height of the tree.
A kite is flying at a height of 60 m above the ground. The string attached to the kite is temporarily tied to a point on the ground. The inclination of the string with the ground is 60°. Find the length of the string, assuming that there is no slack in the string.
The angles of depression of the top and bottom of a tower as seen from the top of a 60 `sqrt(3)` m high cliff are 45° and 60° respectively. Find the height of the tower.
A solid right-circular cone of height 60 cm and radius 30 cm is dropped in a right-circular cylinder full of water of height 180 cm and radius 60 cm. Find the volume of water left in the cylinder, in cubic metres ?
If the height of a vertical pole is 3–√3 times the length of its shadow on the ground, then the angle of elevation of the Sun at that time is
(A) 30°
(B) 60°
(C) 45°
(D) 75°
A solid right circular cone is cut into two parts at the middle of its height by a plane parallel to its base. The ratio of the volume of the smaller cone to the whole cone is
Two buildings are in front of each other on a road of width 15 meters. From the top of the first building, having a height of 12 meter, the angle of elevation of the top of the second building is 30°.What is the height of the second building?
Two buildings are in front of each other on either side of a road of width 10 metres. From the top of the first building which is 20 metres high, the angle of elevation to the top of the second is 45°. What is the height of the second building?
In given figure, the length of AP is ____________.

