Advertisements
Advertisements
प्रश्न
The angles of depression of the top and bottom of a tower as seen from the top of a 60 `sqrt(3)` m high cliff are 45° and 60° respectively. Find the height of the tower.
उत्तर

Let AD be the tower and BC be the cliff.
We have,
BC = 60 `sqrt(3)` , ∠ CDE = 45° and ∠BAC = 60°
Let AD = h
⇒ BE = AD = h
⇒ CE = BC - BE= 60 `sqrt(3)` - h
In ΔCDE,
` tan 45° = (CE)/(DE)`
`⇒ 1 = (60 sqrt(3) -h)/(DE)`
`⇒ DE = 60 sqrt(3) - h`
`⇒ AB = DE = 60 sqrt(3) - h` ............(1)
Now, in ΔABC
`tan 60° = (BC)/(AB)`
`⇒ sqrt(3)= (60 sqrt(3) )/ (60 sqrt(3) -h)` [ Using (1)]
`⇒ 180 - h sqrt(3) = 60 sqrt(3)`
`⇒ h sqrt(3) = 180- 60 sqrt(3)`
`⇒ h = (108 -60sqrt(3) )/sqrt(3) xx sqrt(3)/sqrt(3)`
`⇒ h = ( 180 sqrt(3)-180) /3`
`⇒ h = (180 sqrt(3)-1) /3`
∴` h = 60 ( sqrt(3)-1)`
= 60 (1.732 -1)
= 60 (0.7.32)
Also, h = 43.92m
So, the height of the tower is 43. 92 m.
संबंधित प्रश्न
The angle of elevation of an aeroplane from point A on the ground is 60˚. After flight of 15 seconds, the angle of elevation changes to 30˚. If the aeroplane is flying at a constant height of 1500√3 m, find the speed of the plane in km/hr.
A person observed the angle of elevation of the top of a tower as 30°. He walked 50 m towards the foot of the tower along level ground and found the angle of elevation of the top of the tower as 60°. Find the height of the tower.
A fire in a building B is reported on the telephone to two fire stations P and Q, 20 km apart from each other on a straight road. P observes that the fire is at an angle of 60° to the road and Q observes that it is at an angle of 45° to the road. Which station should send its team and how much will this team have to travel?
Two boats approach a lighthouse in mid-sea from opposite directions. The angles of elevation of the top of the lighthouse from two boats are 30° and 45° respectively. If the distance between two boats is 100 m, find the height of the lighthouse.
An observer 1.5m tall is 30 away from a chimney. The angle of elevation of the top of the chimney from his eye is 60 . Find the height of the chimney.
The horizontal distance between two poles is 15 m. The angle of depression of the top of first pole as seen from the top of second pole is 30°. If the height of the second pole is 24 m, find the height of the first pole. Use`[sqrt3=1.732]`
The angle of elevation of the top of a cell phone tower from the foot of a high apartment is 60° and the angle of depression of the foot of the tower from the top of the apartment is 30°. If the height of the apartment is 50 m, find the height of the cell phone tower. According to radiation control norms, the minimum height of a cell phone tower should be 120 m. State if the height of the above mentioned cell phone tower meets the radiation norms
A bird is sitting on the top of a 80 m high tree. From a point on the ground, the angle of elevation of the bird is 45°. The bird flies away horizontally in such away that it remained at a constant height from the ground. After 2 seconds, the angle of elevation of the bird from the same point is 30°. Determine the speed at which the bird flies `(sqrt(3) = 1.732)`
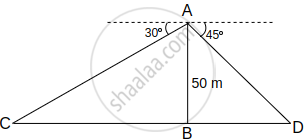
In given figure, the length of AP is ____________.

In the given figure, AB is tower of height 50 m. A man standing on its top, observes two car on the opposite sides of the tower with angles of depression 30° and 45° respectively. Find the distance between the two cars.