Advertisements
Advertisements
प्रश्न
An LR circuit with emf ε is connected at t = 0. (a) Find the charge Q which flows through the battery during 0 to t. (b) Calculate the work done by the battery during this period. (c) Find the heat developed during this period. (d) Find the magnetic field energy stored in the circuit at time t. (e) Verify that the results in the three parts above are consistent with energy conservation.
उत्तर
(a) Let the current in the LR circuit be i.
Let the charge flowing through the coil in the infinitesimal time dt be dq.
Now,
\[i = \frac{dq}{dt}\]
∴ dq = idt
The current in the LR circuit after t seconds after connecting the battery is given by
i = i0 (1 − e−t/τ)
Here,
i0 = Steady state current
τ = Time constant = `L/R`
dq = i0 (1 − e−tR/L) dt
On integrating both sides, we get
\[Q = \int\limits_0^t dq\]
\[= i_0 \left[ \int\limits_0^t dt - \int\limits_0^t e^{- tR/L} dt \right]\]
\[ = i_0 \left[ t - \left( - \frac{L}{R} \right)( e^{- tR/L} - 1) \right]\]
\[ = i_0 \left[ t - \frac{L}{R}(1 - e^{- tR/L} ) \right]\]
Thus, the charge flowing in the coil in time t is given by
\[Q = \frac{\epsilon}{R}\left[ t - \frac{L}{R}(1 - e^{- tR/L} ) \right]\]
Where `i_0=epsilon/R`
(b) The work done by the battery is given by
W = εQ
From the above expression for the charge in the LR circuit, we have
\[W = \frac{\epsilon^2}{R}\left[ t - \frac{L}{R}(1 - e^{- tR/L} ) \right]\]
(c) The heat developed in time t can be calculated as follows:-
\[H = \int\limits_0^t i^2 Rdt\]
\[H = \frac{\epsilon^2}{R^2}R \int\limits_0^t (1 - e^{- tR/L} )^2 dt\]
\[H = \frac{\epsilon^2}{R} \int\limits_0^t \left[ (1 + e^{- 2tR/L} ) - 2 e^{- tR/L} \right]dt\]
\[H = \frac{\epsilon^2}{R} \left( t - \frac{L}{2R} e^{- 2tR/L} + \frac{L}{R}2 e^{- tR/L} \right)_0^t \]
\[H = \frac{\epsilon^2}{R}\left( t - \frac{L}{2R} e^{- 2tR/L} + \frac{L}{R}2 e^{- tR/L} \right) - \left( - \frac{L}{2R} + \frac{2L}{R} \right)\]
\[ = \frac{\epsilon^2}{R}\left\{ t - \frac{L}{2R}( x^2 - 4x + 3) \right\}...........\left(x = e^{- tR/L}\right)\]
(d) The magnetic energy stored in the circuit is given by
\[U = \frac{1}{2}L i^2 \]
\[ \Rightarrow U = \frac{1}{2}L\frac{\epsilon^2}{R^2} \left(1 - e^{- tR/L}\right)^2 \]
\[\Rightarrow U = \frac{L \epsilon^2}{2 R^2}(1 - x )^2\]
(e) Taking the sum of total energy stored in the magnetic field and the heat developed in time t
\[E = \frac{L \epsilon^2}{2 R^2}\left(1 - x\right)^2 + \frac{\epsilon^2}{R}\left\{ t - \frac{L}{2R}\left(x^2 - 4x + 3\right) \right\}\]
\[E = \frac{L \epsilon^2}{2 R^2}\left(1 + x^2 - 2x\right) + \frac{\epsilon^2}{R}t - \frac{L \epsilon^2}{2 R^2}\left(x^2 - 4x + 3\right)\]
\[E = \frac{L \epsilon^2}{2 R^2}\left(2x - 2\right) + \frac{\epsilon^2}{R}t\]
\[E = \frac{L \epsilon^2}{R^2}\left(x - 1\right) + \frac{\epsilon^2}{R}t\]
\[E = \frac{\epsilon^2}{R}\left\{ t - \frac{L}{R}\left(1 - x\right) \right\} = \frac{\epsilon^2}{R}\left\{ t - \frac{L}{R}\left(1 - e^{- tR/L}\right) \right\}\]
The above expression is equal to the energy drawn from the battery. Therefore, the conservation of energy holds good.
APPEARS IN
संबंधित प्रश्न
The figure shows a series LCR circuit with L = 10.0 H, C = 40 μF, R = 60 Ω connected to a variable frequency 240 V source, calculate
(i) the angular frequency of the source which drives the circuit at resonance,
(ii) the current at the resonating frequency,
(iii) the rms potential drop across the inductor at resonance.
Show that in an a.c. circuit containing a pure inductor, the voltage is ahead of current by π/2 in phase ?
The current in a discharging LR circuit without the battery drops from 2.0 A to 1.0 A in 0.10 s. (a) Find the time constant of the circuit. (b) If the inductance of the circuit 4.0 H, what is its resistance?
(i) An a.c. source of emf ε = 200 sin omegat is connected to a resistor of 50 Ω . calculate :
(1) Average current (`"I"_("avg")`)
(2) Root mean square (rms) value of emf
(ii) State any two characteristics of resonance in an LCR series circuit.
The potential difference across the resistor is 160V and that across the inductor is 120V. Find the effective value of the applied voltage. If the effective current in the circuit be 1.0 A, calculate the total impedance of the circuit.
What will be the potential difference in the circuit when direct current is passed through the circuit?
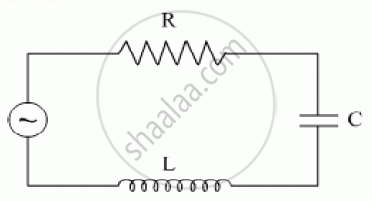
In a series, LCR circuit, obtain an expression for the resonant frequency,
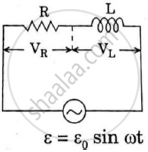
Using the phasor diagram, derive the expression for the current flowing in an ideal inductor connected to an a.c. source of voltage, v= vo sin ωt. Hence plot graphs showing the variation of (i) applied voltage and (ii) the current as a function of ωt.
Derive an expression for the average power dissipated in a series LCR circuit.
A series LCR circuit with R = 20 Ω, L = 1.5 H and C = 35 µF is connected to a variable-frequency 200 V ac supply. When the frequency of the supply equals the natural frequency of the circuit, what is the average power transferred to the circuit in one complete cycle?
In an L.C.R. series a.c. circuit, the current ______.
To reduce the resonant frequency in an LCR series circuit with a generator
A series LCR circuit containing 5.0 H inductor, 80 µF capacitor and 40 Ω resistor is connected to 230 V variable frequency ac source. The angular frequencies of the source at which power transferred to the circuit is half the power at the resonant angular frequency are likely to be ______.
To reduce the resonant frequency in an LCR series circuit with a generator ______.
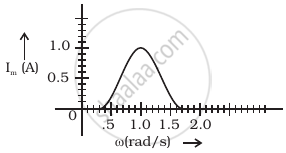
In series LCR circuit, the plot of Imax vs ω is shown in figure. Find the bandwidth and mark in the figure.
An alternating voltage of 220 V is applied across a device X. A current of 0.22 A flows in the circuit and it lags behind the applied voltage in phase by π/2 radian. When the same voltage is applied across another device Y, the current in the circuit remains the same and it is in phase with the applied voltage.
- Name the devices X and Y and,
- Calculate the current flowing in the circuit when the same voltage is applied across the series combination of X and Y.
A series LCR circuit is connected to an ac source. Using the phasor diagram, derive the expression for the impedance of the circuit.
Three students, X, Y and Z performed an experiment for studying the variation of a.c. with frequency in a series LCR circuit and obtained the graphs as shown below. They all used
- an AC source of the same emf and
- inductance of the same value.
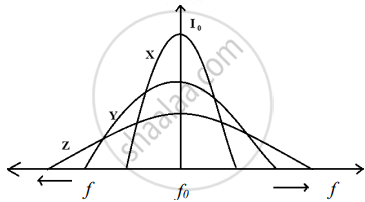
- Who used minimum resistance?
- In which case will the quality Q factor be maximum?
- What did the students conclude about the nature of impedance at resonant frequency (f0)?
- An ideal capacitor is connected across 220V, 50Hz, and 220V, 100Hz supplies. Find the ratio of current flowing through it in the two cases.
