Advertisements
Advertisements
प्रश्न
Classically, an electron can be in any orbit around the nucleus of an atom. Then what determines the typical atomic size? Why is an atom not, say, a thousand times bigger than its typical size? The question had greatly puzzled Bohr before he arrived at his famous model of the atom that you have learnt in the text. To simulate what he might well have done before his discovery, let us play as follows with the basic constants of nature and see if we can get a quantity with the dimensions of length that is roughly equal to the known size of an atom (~ 10−10 m).
(a) Construct a quantity with the dimensions of length from the fundamental constants e, me, and c. Determine its numerical value.
(b) You will find that the length obtained in (a) is many orders of magnitude smaller than the atomic dimensions. Further, it involves c. But energies of atoms are mostly in non-relativistic domain where c is not expected to play any role. This is what may have suggested Bohr to discard c and look for ‘something else’ to get the right atomic size. Now, the Planck’s constant h had already made its appearance elsewhere. Bohr’s great insight lay in recognising that h, me, and e will yield the right atomic size. Construct a quantity with the dimension of length from h, me, and e and confirm that its numerical value has indeed the correct order of magnitude.
उत्तर
(a) Charge on an electron, e = 1.6 × 10−19 C
Mass of an electron, me = 9.1 × 10−31 kg
Speed of light, c = 3 × 108 m/s
Let us take a quantity involving the given quantities as `("e"^2/(4piin_0 "m"_"e""c"^2))`.
Where,
∈0 = Permittivity of free space
And `1/(4pi in_0)` = 9 × 109 N m2 C−2
The numerical value of the taken quantity will be:
`1/(4pi in_0) xx "e"^2/("m"_"e""c"^2)`
= `9 xx 10^9 xx (1.6 xx 10^(-19))^2/(9.1 xx 10^(-31) xx (3 xx 10^8)^2`
= 2.81 × 10−15 m
Hence, the numerical value of the taken quantity is much smaller than the typical size of an atom.
(b) Charge on an electron, e = 1.6 × 10−19 C
Mass of an electron, me = 9.1 × 10−31 kg
Planck’s constant, h = 6.63 × 10−34 Js
Let us take a quantity involving the given quantities as `(4pi in_0 ("h"/(2pi))^2)/("m"_"e" "e"^2)`.
Where,
∈0 = Permittivity of free space
And , `1/(4pi in_0)` = 9 × 109 N m2 C−2
The numerical value of the taken quantity will be:
`4pi in_0 xx ("h"/(2pi))^2/("m"_"e""e"^2)`
= `1/ (9 xx 10^9) xx ((6.63 xx 10^(-34))/(2 xx 3.14))^2/(9.1 xx 10^-31 xx (1.6 xx 10^-19)^2)`
= 0.53 × 10−10 m
Hence, the value of the quantity taken is of the order of the atomic size.
APPEARS IN
संबंधित प्रश्न
A 12.5 eV electron beam is used to bombard gaseous hydrogen at room temperature. What series of wavelengths will be emitted?
The minimum orbital angular momentum of the electron in a hydrogen atom is
In which of the following transitions will the wavelength be minimum?
Which of the following curves may represent the speed of the electron in a hydrogen atom as a function of trincipal quantum number n?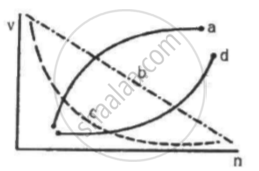
The radius of the shortest orbit in a one-electron system is 18 pm. It may be
Find the radius and energy of a He+ ion in the states (a) n = 1, (b) n = 4 and (c) n = 10.
A hydrogen atom emits ultraviolet radiation of wavelength 102.5 nm. What are the quantum numbers of the states involved in the transition?
A hydrogen atom in a state having a binding energy of 0.85 eV makes transition to a state with excitation energy 10.2 e.V (a) Identify the quantum numbers n of the upper and the lower energy states involved in the transition. (b) Find the wavelength of the emitted radiation.
Whenever a photon is emitted by hydrogen in Balmer series, it is followed by another photon in Lyman series. What wavelength does this latter photon correspond to?
Suppose, in certain conditions only those transitions are allowed to hydrogen atoms in which the principal quantum number n changes by 2. (a) Find the smallest wavelength emitted by hydrogen. (b) List the wavelength emitted by hydrogen in the visible range (380 nm to 780 nm).
Average lifetime of a hydrogen atom excited to n = 2 state is 10−8 s. Find the number of revolutions made by the electron on the average before it jumps to the ground state.
A hydrogen atom in ground state absorbs a photon of ultraviolet radiation of wavelength 50 nm. Assuming that the entire photon energy is taken up by the electron with what kinetic energy will the electron be ejected?
Electrons are emitted from an electron gun at almost zero velocity and are accelerated by an electric field E through a distance of 1.0 m. The electrons are now scattered by an atomic hydrogen sample in ground state. What should be the minimum value of E so that red light of wavelength 656.3 nm may be emitted by the hydrogen?
A hydrogen atom moving at speed υ collides with another hydrogen atom kept at rest. Find the minimum value of υ for which one of the atoms may get ionized.
The mass of a hydrogen atom = 1.67 × 10−27 kg.
When a photon is emitted from an atom, the atom recoils. The kinetic energy of recoil and the energy of the photon come from the difference in energies between the states involved in the transition. Suppose, a hydrogen atom changes its state from n = 3 to n = 2. Calculate the fractional change in the wavelength of light emitted, due to the recoil.
The Balmer series for the H-atom can be observed ______.
- if we measure the frequencies of light emitted when an excited atom falls to the ground state.
- if we measure the frequencies of light emitted due to transitions between excited states and the first excited state.
- in any transition in a H-atom.
- as a sequence of frequencies with the higher frequencies getting closely packed.
Let En = `(-1)/(8ε_0^2) (me^4)/(n^2h^2)` be the energy of the nth level of H-atom. If all the H-atoms are in the ground state and radiation of frequency (E2 - E1)/h falls on it ______.
- it will not be absorbed at all.
- some of atoms will move to the first excited state.
- all atoms will be excited to the n = 2 state.
- no atoms will make a transition to the n = 3 state.
Positronium is just like a H-atom with the proton replaced by the positively charged anti-particle of the electron (called the positron which is as massive as the electron). What would be the ground state energy of positronium?
A hydrogen atom makes a transition from n = 5 to n = 1 orbit. The wavelength of photon emitted is λ. The wavelength of photon emitted when it makes a transition from n = 5 to n = 2 orbit is ______.
