Advertisements
Advertisements
प्रश्न
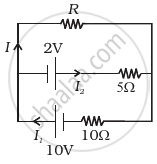
Consider the circuit shown in the figure. Find (a) the current in the circuit (b) the potential drop across the 5 Ω resistor (c) the potential drop across the 10 Ω resistor (d) Answer the parts (a), (b) and (c) with reference to the figure.


उत्तर
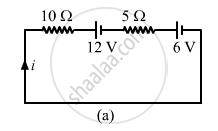
(a)
Applying KVL in the above loop, we get:-
\[10i + 6 + 5i + 12 = 0\]
\[ \Rightarrow 10i + 5i = - 18\]
\[ \Rightarrow 15i = - 18\]
\[ \Rightarrow i = - \frac{18}{15} = - \frac{6}{5} = - 1 . 2 A\]
The negative sign indicates that current is flowing in the direction opposite to our assumed direction.
(b) Potential drop across the 5 Ω resistor= 5i = 5×(-1.2 ) = -6 V
(c) Potential drop across the 10 Ω resistor = 10i = (-1.2) × 10 = 12 V
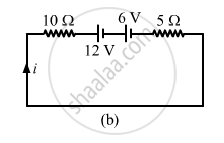
(d)
Applying KVL in the above loop, we get:-
\[10i + 5i + 6 + 12 = 0\]
\[ \Rightarrow 15i = - 18\]
\[ \Rightarrow i = - 1 . 2 A\]
Potential drop across the 5Ω register = -6 V
Potential drop across the 10Ω register = -12 V
APPEARS IN
संबंधित प्रश्न
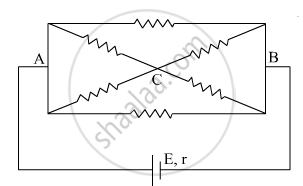
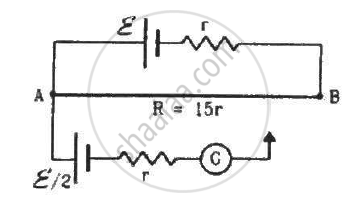
The current is drawn from a cell of emf E and internal resistance r connected to the network of resistors each of resistance r as shown in the figure. Obtain the expression for
- the current draw from the cell and
- the power consumed in the network.
Given the resistances of 1 Ω, 2 Ω, 3 Ω, how will be combine them to get an equivalent resistance of (11/3) Ω?
Given the resistances of 1 Ω, 2 Ω, 3 Ω, how will be combine them to get an equivalent resistance of (6/11) Ω?
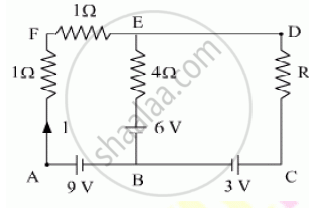
Using Kirchhoff’s rules determine the value of unknown resistance R in the circuit so that no current flows through 4 Ω resistance. Also find the potential difference between A and D.
Consider the potentiometer circuit as arranged in the figure. The potentiometer wire is 600 cm long. (a) At what distance from the point A should the jockey touch the wire to get zero deflection in the galvanometer? (b) If the jockey touches the wire at a distance of 560 cm from A, what will be the current in the galvanometer?
Twelve wires each having a resistance of 3 Ω are connected to form a cubical network. A battery of 10 V and negligible internal resistance is connected across the diagonally opposite corners of this network. Determine its equivalent resistance and the current along each edge of the cube.
State Kirchhoff ’s voltage rule.
How the emf of two cells are compared using potentiometer?
Lightning is a very good example of a natural current. In typical lightning, there is 109 J energy transfer across the potential difference of 5 × 107 V during a time interval of 0.2 s. Using this information, estimate the following quantities:
- the total amount of charge transferred between cloud and ground
- the current in the lightning bolt
- the power delivered in 0.2 s.

In a potentiometer arrangement, a cell of emf 1.25 V gives a balance point at 35 cm length of the wire. If the cell is replaced by another cell and the balance point shifts to 63 cm, what is the emf of the second cell?
The instrument for the accurate measurement of the e.m.f of a cell is ______.
Kirchhoff’s second law is a consequence of law of conservation of ______.
Two cell of 1.25 V and 0.75 V are connected parallel. The effective voltage will be:-
What is the advantage of using thick metallic strips to join wires in a potentiometer?
Two cells of voltage 10V and 2V and internal resistances 10Ω and 5Ω respectively, are connected in parallel with the positive end of 10V battery connected to negative pole of 2V battery (Figure). Find the effective voltage and effective resistance of the combination.
State the two Kirchhoff’s rules used in the analysis of electric circuits and explain them.
Derive the equation of the balanced state in a Wheatstone bridge using Kirchhoff’s laws.
In the circuit shown in Figure below, E1 and E2 are batteries having emfs of 25V and 26V. They have an internal resistance of 1 Ω and 5 Ω respectively. Applying Kirchhoff’s laws of electrical networks, calculate the currents I1 and I2.

