Advertisements
Advertisements
प्रश्न
Consider the following very rough model of a beryllium atom. The nucleus has four protons and four neutrons confined to a small volume of radius 10−15 m. The two 1 selectrons make a spherical charge cloud at an average distance of 1⋅3 ×10−11 m from the nucleus, whereas the two 2 s electrons make another spherical cloud at an average distance of 5⋅2 × 10−11 m from the nucleus. Find three electric fields at (a) a point just inside the 1 s cloud and (b) a point just inside the 2 s cloud.
उत्तर
(a)
Let us consider the three surfaces as three concentric spheres A, B and C.
Let us take q = 1.6× 10-19 C .
Sphere A is the nucleus; so, the charge on sphere A, q1 = 4q
Sphere B is the sphere enclosing the nucleus and the 2 1s electrons; so charge on this sphere, q2 = 4q -2q = 2q
Sphere C is the sphere enclosing the nucleus and the 4 electrons of Be; so, the charge enclosed by this sphere,
q3 = 4q -4q = 0
Radius of sphere A, r1 = 10-15 m
Radius of sphere B, r2 = 1.3 × 10-11 m
Radius of sphere C, r3 = 5.2 × 10-11 m
As the point 'P' is just inside the spherical cloud 1s, its distance from the centre
x = 1.3 × 10-11 m
Electric field,
`"E" = q/(4 pi ∈_0 "x"^2)`
Here, the charge enclosed is due to the charge of the 4 protons inside the nucleus . So,
`"E" = (4 xx (1.6 xx 10^-19))/(4 xx 3.14 xx (8.85 xx 10^-12)xx ( 1.3 xx 10^-11)^2 ) `
E = 3.4× 1013 N/C
(b)
For a point just inside the 2s cloud, the total charge enclosed will be due to the 4 protons and 2 electrons. Charge enclosed,
qen = 2q = 2× (1.6 ×10-19) C
Hence, electric field,
`"E" = ("q"_"en")/ (4 pi ∈_0"x"^2)`
x = 5.2× 10-11 m
`"E" = (2 xx (1.6 xx 10^-19))/(4 xx 3.14 xx ( 8.85 xx 10^-12) xx ( 5.2 xx 10^-11)^2)`
E = 1.065 × 1012 N/C
Thus , E =1.1× 1012 N/C
APPEARS IN
संबंधित प्रश्न
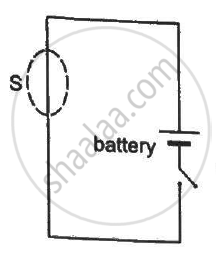
A closed surface S is constructed around a conducting wire connected to a battery and a switch in the following figure. As the switch is closed, the free electrons in the wire start moving along the wire. In any time interval, the number of electrons entering the closed surface S is equal to the number of electrons leaving it. On closing the switch, the flux of the electric field through the closed surface
(a) is increased
(b) is decreased
(c) remains unchanged
(d) remains zero
A charge Q is uniformly distributed over a rod of length l. Consider a hypothetical cube of edge l with the centre of the cube at one end of the rod. Find the minimum possible flux of the electric field through the entire surface of the cube.
The electric field in a region is given by
`vec"E"= 3/5"E"_0 vec"i" + 4/5 "E"_0 vec "i" "with" " E"_0 = 2.0 xx 10^3 "N""C"^-1.`
Find the flux of this field through a rectangular surface of area 0⋅2 m2 parallel to the y-z plane.
The electric field in a region is given by `vec"E" = ("E"_0 "x")/"l" vec"i".`
Find the charge contained inside the cubical volume bound by the surfaces
x =0, x =a, y=0, y=a, z=0 and z=a. Take
`"E"_0 = 5 xx 10^3 "N""C"^-1 , "l" =2 "cm" " and" " a" = 1 "cm" `
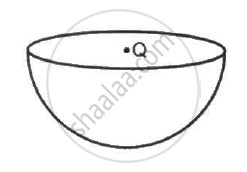
A charge Q is placed at the centre of an imaginary hemispherical surface. Using symmetry arguments and Gauss's Law, find the flux of the electric field due to this charge through the surface of the hemisphere in the following figure.
A charge Q is placed at the centre of an uncharged, hollow metallic sphere of radius a. (a) Find the surface. (b) If a charge q is put on the sphere, what would be the surface charge densities on the inner and outer surfaces? (c) Find the electric field inside the sphere at a distance x from the centre in the situations (a) and (b).
Find the magnitude of the electric field at a point 4 cm away from a line charge of density 2 × 10-6 Cm-1.
A long cylindrical wire carries a positive charge of linear density 2.0 × 10-8 C m -1 An electron revolves around it in a circular path under the influence of the attractive electrostatic force. Find the kinetic energy of the electron. Note that it is independent of the radius.
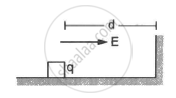
A block of mass containing a net positive charge q is placed on a smooth horizontal table which terminates in a vertical wall as shown in the figure. The distance of the block from the wall is d. A horizontal electric field E towards the right is switched on. Assuming elastic collisions (if any), find the time period of the resulting oscillatory motion. Is it a simple harmonic motion?
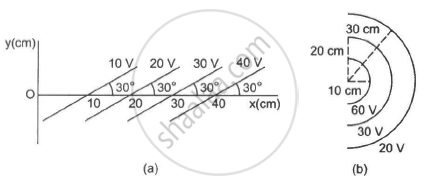
Some equipotential surface is shown in the figure. What can you say about the magnitude and the direction of the electric field?
An electric field of magnitude 1000 NC−1 is produced between two parallel plates with a separation of 2.0 cm, as shown in the figure. (a) What is the potential difference between the plates? (b) With what minimum speed should an electron be projected from the lower place in the direction of the field, so that it may reach the upper plate? (c) Suppose the electron is projected from the lower place with the speed calculated in part (b). The direction of projection makes an angle of 60° with the field. Find the maximum height reached by the electron.
A uniform field of 2.0 NC−1 exists in space in the x-direction. (a) Taking the potential at the origin to be zero, write an expression for the potential at a general point (x, y, z). (b) At which point, the potential is 25 V? (c) If the potential at the origin is taken to be 100 V, what will be the expression for the potential at a general point? (d) What will be the potential at the origin if the potential at infinity is taken to be zero? Is it practical to choose the potential at infinity to be zero?
Answer the following question.
Prove that the average energy density of the oscillating electric field is equal to that of the oscillating magnetic field.
Electric field at a point is defined as ______.
Two charged conducting spheres of radii a and b are connected to each other by a wire. Find the ratio of the electric fields at their surfaces.
