Advertisements
Advertisements
प्रश्न
Construct an isosceles triangle whose base is 8 cm and altitude 4 cm and then another triangle whose side are `1 1/2` times the corresponding sides of the isosceles triangle.
Give the justification of the construction
उत्तर
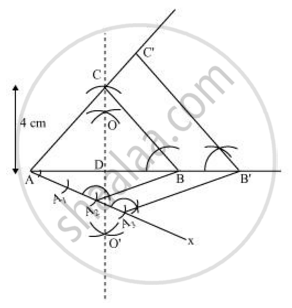
Let us assume that ΔABC is an isosceles triangle having CA and CB of equal lengths, base AB of 8 cm, and AD is the altitude of 4 cm.
A ΔAB'C' whose sides are 3/2 times of ΔABC can be drawn as follows.
Step 1
Draw a line segment AB of 8 cm. Draw arcs of same radius on both sides of the line segment while taking point A and B as its centre. Let these arcs intersect each other at O and O'. Join OO'. Let OO' intersect AB at D.
Step 2
Taking D as centre, draw an arc of 4 cm radius which cuts the extended line segment OO' at point C. An isosceles ΔABC is formed, having CD (altitude) as 4 cm and AB (base) as 8 cm.
Step 3
Draw a ray AX making an acute angle with line segment AB on the opposite side of vertex C.
Step 4
Locate 3 points (as 3 is greater between 3 and 2) A1, A2, and A3 on AX such that AA1 = A1A2 = A2A3.
Step 5
Join BA2 and draw a line through A3 parallel to BA2 to intersect extended line segment AB at point B'.
Step 6
Draw a line through B' parallel to BC intersecting the extended line segment AC at C'. ΔAB'C' is the required triangle.
Justification
The construction can be justified by proving that
`AB' = 3/2 AB, B'C' = 3/2 BC, AC' = 3/2 AC`
In ΔABC and ΔAB'C',
∠ABC = ∠AB'C' (Corresponding angles)
∠BAC = ∠B'AC' (Common)
∴ ΔABC ∼ ΔAB'C' (AA similarity criterion)
`=> (AB)/(AB')= (BC)/(B'C') = (AC)/(AC') ....(1)`
In ΔAA2B and ΔAA3B',
∠A2AB = ∠A3AB' (Common)
∠AA2B = ∠AA3B' (Corresponding angles)
∴ ΔAA2B ∼ ΔAA3B' (AA similarity criterion)
`=> (AB)/(AB') = (`
`=>(AB)/(AB') = 2/3 .....2`
On comparing equations (1) and (2), we obtain
`(AB)/(AB')=(BC)/(B'C') = (AC)/(AC') = 2/3`
`=> AB' = 3/2 AB, B'C' = 3/2 BC, AC' = 3/2 AC`
This justifies the construction.
APPEARS IN
संबंधित प्रश्न
Write down the equation of a line whose slope is 3/2 and which passes through point P, where P divides the line segment AB joining A(-2, 6) and B(3, -4) in the ratio 2 : 3.
Draw a right triangle in which the sides (other than the hypotenuse) are of lengths 4 cm and 3 cm. Now construct another triangle whose sides are `3/5` times the corresponding sides of the given triangle.
Divide a line segment of length 9 cm internally in the ratio 4 : 3. Also, give justification of the construction.
Construct a triangle similar to a given ΔXYZ with its sides equal to (3/4)th of the corresponding sides of ΔXYZ. Write the steps of construction.
Find the ratio in which point T(–1, 6)divides the line segment joining the points P(–3, 10) and Q(6, –8).
Δ AMT ∼ ΔAHE. In Δ AMT, MA = 6.3 cm, ∠MAT = 120°, AT = 4.9 cm, `(MA)/(HA) = 7/5`. construct Δ AHE.
Draw seg AB of length 9 cm and divide it in the ratio 3 : 2
To divide a line segment PQ in the ratio 5 : 7, first a ray PX is drawn so that ∠QPX is an acute angle and then at equal distances points are marked on the ray PX such that the minimum number of these points is ______.
What is the ratio `(AC)/(BC)` for the line segment AB following the construction method below?
Step 1: A ray is extended from A and 30 arcs of equal lengths are cut, cutting the ray at A1, A2,…A30
Step 2: A line is drawn from A30 to B and a line parallel to A30B is drawn, passing through the point A17 and meet AB at C.
Draw a line segment of length 7 cm and divide it in the ratio 5 : 3.
