Advertisements
Advertisements
प्रश्न
Depict the equipotential surface due to
(i) an electric dipole,
(ii) two identical positive charges separated by a distance.
उत्तर
Equipotential surfaces for (a) a dipole and (b) two identical positive charges are shown in Figure.
Notes
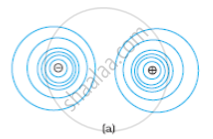
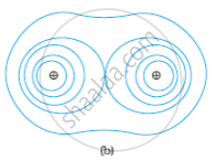
Some equipotential surfaces for (a) a dipole, (b) two identical positive charges.
APPEARS IN
संबंधित प्रश्न
Draw a sketch of equipotential surfaces due to a single charge (-q), depicting the electric field lines due to the charge
A regular hexagon of side 10 cm has a charge 5 µC at each of its vertices. Calculate the potential at the centre of the hexagon.
Draw equipotential surfaces:
(1) in the case of a single point charge and
(2) in a constant electric field in Z-direction. Why are the equipotential surfaces about a single charge not equidistant?
(3) Can electric field exist tangential to an equipotential surface? Give reason
Find the amount of work done in rotating an electric dipole of dipole moment 3.2 x 10- 8Cm from its position of stable equilibrium to the position of unstable equilibrium in a uniform electric field if intensity 104 N/C.
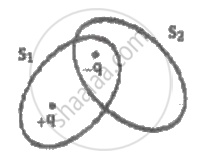
S1 and S2 are the two imaginary surfaces enclosing the charges +q and -q as shown. The electric flux through S1 and S2 are respectively ______.
Equipotentials at a great distance from a collection of charges whose total sum is not zero are approximately.
- The potential at all the points on an equipotential surface is same.
- Equipotential surfaces never intersect each other.
- Work done in moving a charge from one point to other on an equipotential surface is zero.
The work done to move a charge along an equipotential from A to B ______.
- cannot be defined as `- int_A^B E.dl`
- must be defined as `- int_A^B E.dl`
- is zero.
- can have a non-zero value.
Prove that a closed equipotential surface with no charge within itself must enclose an equipotential volume.
What is meant by an equipotential surface?
