Advertisements
Advertisements
प्रश्न
Derive the expression for the magnetic field due to a current-carrying coil of radius r at a distance x from the center along the X-axis.
उत्तर
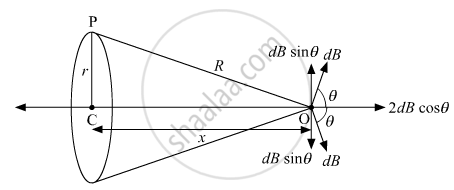
Consider a conducting element dl of the loop. The magnitude dB of the magnetic field due to dl is given by the Biot-Savart law,
`"dB" = mu_°/(4pi) ("i"|vec(dl) xx vec("R")|)/"R"^3 `
`"dB" = mu_° /(4pi) ("idl")/"R"^2 (vec"idl" ⊥ vec"R")`
From the above figure, we can see, R2 = r2 +x2
`"dB" = mu_° /(4pi) xx "idl"/("r"^2 + "x"^2)`
The direction of the magnetic field is shown in the figure, as we can see, only cosine component of the magnetic field will play a role here, all the sine component will get cancel out to give a zero net value.
`"dB"_"net" = mu_°/(4pi) xx "idl"/(("r"^2 + "x"^2)) xx costheta`
`"dB"_"net" = mu_°/(4pi) xx "idl"/(("r"^2 + "x"^2)) xx "r"/(sqrt("r"^2+"x"^2)) `
`"dB"_"net" = (mu_° "idl")/(4pi) "r"/(("r"^2 + "x"^2)^(3//2))`
∵ dl = rdθ
⇒ `"B"_"net" = (mu_°"i")/(4pi) ("r"^2 int_0^(2pi) "d"theta)/(("r"^2 + "x"^2)^(3//2)) = (mu_°"ir"^2)/(2("r"^2+ "x"^2)^(3//2))`
⇒ `"B"_"net" = (mu_°"ir"^2)/(2("r"^2+ "x"^2)^(3//2))`
⇒ `vec("B"_"net") = (mu_°"ir"^2)/(2("r"^2+ "x"^2)^(3//2)) hat"i"`
APPEARS IN
संबंधित प्रश्न
Using Biot − Savart’s law, derive the expression for the magnetic field in the vector form at a point on the axis of a circular current loop?
What does a toroid consist of? Find out the expression for the magnetic field inside a toroid for N turns of the coil having the average radius r and carrying a current I. Show that the magnetic field in the open space inside and exterior to the toroid is zero.
The magnetic field at the origin due to a current element \[i d \vec{l}\] placed at a position \[\vec{r}\] is
(a)\[\frac{\mu_0 i}{4\pi}\frac{d \vec{l} \times \vec{r}}{r^3}\]
(b) \[- \frac{\mu_0 i}{4\pi}\frac{\vec{r} \times d \vec{l}}{r^3}\]
(c) \[\frac{\mu_0 i}{4\pi}\frac{\vec{r} \times d \vec{l}}{r^3}\]
(d) \[- \frac{\mu_0 i}{4\pi}\frac{d \vec{l} \times \vec{r}}{r^3}\]
A wire of length l is bent in the form of an equilateral triangle and carries an electric current i. (a) Find the magnetic field B at the centre. (b) If the wire is bent in the form of a square, what would be the value of B at the centre?
A wire of length l is bent in the form of an equilateral triangle and carries an electric current i. Find the magnetic field B at the centre.
An electric current I flows through a circular loop as shown in Figure 2(b) below. Write an expression and direction for the magnetic field at the centre of the loop at point P.
State Biot Savart law.
A circular loop of radius 0.3 cm lies parallel to much bigger circular of radius 20 cm. The centre of the small loop is on the axis of the bigger loop. The distance between their centres is 15 cm. If a current of 2.0 A flows through the smaller loop, then the flux linked with the bigger loop is ______.
The magnetic field at any point on the axis of a current element is ______
Two long wires carrying current I1 and I2 are arranged as shown in figure. The one carrying current I1 is along is the x-axis. The other carrying current I2 is along a line parallel to the y-axis given by x = 0 and z = d. Find the force exerted at O2 because of the wire along the x-axis.

