Advertisements
Advertisements
प्रश्न
Draw a pair of tangents to a circle of radius 3 cm, which are inclined to each other at an angle of 60°.
उत्तर
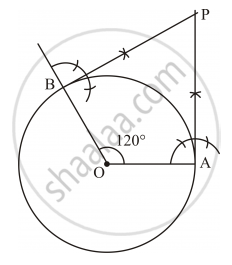
The tangents can be constructed in the following manner:
(i) Draw a circle of radius 3 cm with the centre as O.
(ii) Take a point A on the circumference of the circle and join OA. Draw a perpendicular to OA at point A.
(iii) Draw a radius OB, making an angle of 120° [(180° − 60°)] with OA.
(iv) Draw a perpendicular to OB at point B. Let both the perpendiculars intersect at point P.
PA and PB are the required tangents at an angle of 60°.
APPEARS IN
संबंधित प्रश्न
Construct a tangent to a circle of radius 4 cm from a point on the concentric circle of radius 6 cm and measure its length. Also verify the measurement by actual calculation.
In the given figure PQ is a tangent to the circle at A, AB and AD are bisectors of `angleCAQ` and `angle PAC`. if `angleBAQ = 30^@. prove that:
1) BD is a diameter of the circle
2) ABC is an isosceles triangle

Draw a circle of diameter 9 cm. Mark a point at a distance of 7.5 cm from the centre of the circle. Draw tangents to the given circle from this exterior point. Measure the length of each tangent.
Using ruler and compasses only, draw an equilateral triangle of side 4.5 cm and draw its circumscribed circle. Measure the radius of the circle.
Draw a circle of radius 4.5 cm. Draw two tangents to this circle so that the angle between the tangents is 60°.
Draw a circle of radius 3 cm. Form a point P, 7 cm away from the centre of the circle, draw two tangents to the circle. Also, measure the lengths of the tangents
Draw a circle of radius 32 cm. Draw a tangent to the circle making an angle 30° with a line passing through the centre.
Use rule and compass only for answering this question.
Draw a circle of radius 4 cm. Mark the centre as O. Mark a point P outside the circle at a distance of 7 cm from the centre. Construct two tangents to the circle from the external point P.
Measure and write down the length of any one tangent.
Draw a circle with centre O and radius 2.5 cm. Take a point P at a distance of 6 cm from the centre. Using ruler and compasses only construct the tangents to the circle from the point P.
In Figure 2, PQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q intersect at a point T. Find the length TP.

