Advertisements
Advertisements
प्रश्न
Draw ∆PQR such that PQ = 6.8 cm, vertical angle is 50° and the bisector of the vertical angle meets the base at D where PD = 5.2 cm
उत्तर
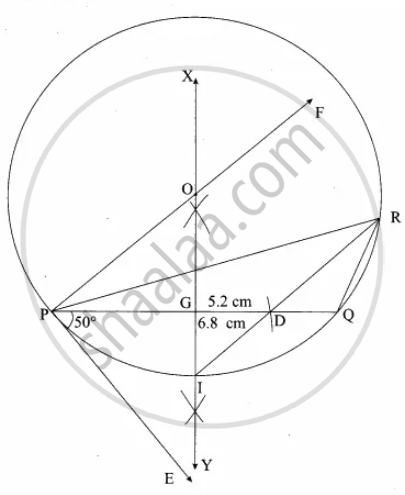
Steps of construction:
1. Draw a line segment PQ = 6.8 cm.
2. At P draw PE such that ∠QPE = 50°.
3. At P draw PF such that ∠EPF = 90°.
4. Draw the perpendicular bisector to PQ which intersects PF at O and PQ at G.
5. With O as centre and OP as radius draw a circle.
6. From P mark an arc of 5.2 cm on PQ at D.
7. The perpendicular bisector intersects the circle at I. Join ID.
8. ID produced meets the circle at A. Now Joint PR and QR. This ∆PQR is the required triangle.
APPEARS IN
संबंधित प्रश्न
In ∆ABC, D and E are points on the sides AB and AC respectively such that DE || BC
If `"AD"/"DB" = 3/4` and AC = 15 cm find AE
In ∆ABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 8x – 7, DB = 5x – 3, AE = 4x – 3 and EC = 3x – 1, find the value of x
In ΔABC, D and E are points on the sides AB and AC respectively. For the following case show that DE || BC
AB = 12 cm, AD = 8 cm, AE = 12 cm and AC = 18 cm
If PQ || BC and PR || CD prove that `"AR"/"AD" = "AQ"/"AB"`

Rhombus PQRB is inscribed in ΔABC such that ∠B is one of its angle. P, Q and R lie on AB, AC and BC respectively. If AB = 12 cm and BC = 6 cm, find the sides PQ, RB of the rhombus.
ABCD is a quadrilateral in which AB = AD, the bisector of ∠BAC and ∠CAD intersect the sides BC and CD at the points E and F respectively. Prove that EF || BD.
Construct a ∆PQR in which QR = 5 cm, ∠P = 40° and the median PG from P to QR is 4.4 cm. Find the length of the altitude from P to QR.
ABC is a triangle in which AB = AC. Points D and E are points on the side AB and AC respectively such that AD = AE. Show that the points B, C, E and D lie on a same circle
An Emu which is 8 feet tall is standing at the foot of a pillar which is 30 feet high. It walks away from the pillar. The shadow of the Emu falls beyond Emu. What is the relation between the length of the shadow and the distance from the Emu to the pillar?
Two circles intersect at A and B. From a point, P on one of the circles lines PAC and PBD are drawn intersecting the second circle at C and D. Prove that CD is parallel to the tangent at P.
