Advertisements
Advertisements
प्रश्न
Draw seg AB = 6.8 cm and draw perpendicular bisector of it.
उत्तर

APPEARS IN
संबंधित प्रश्न
Given below is the triangle and length of line segments. Identify in the given figure, ray PM is the bisector of ∠QPR.

Given below is the triangle and length of line segments. Identify in the given figure, ray PM is the bisector of ∠QPR.

In ∆MNP, NQ is a bisector of ∠N. If MN = 5, PN = 7 MQ = 2.5 then find QP.

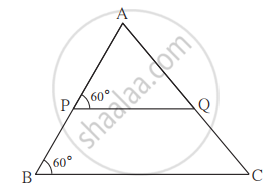
Measures of some angles in the figure are given. Prove that `"AP"/"PB" = "AQ"/"QC"`.
Find QP using given information in the figure.

In the given figure, if AB || CD || FE then find x and AE.

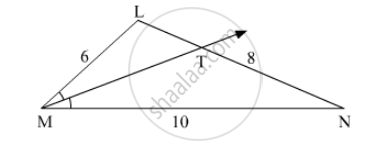
In ∆LMN, ray MT bisects ∠LMN If LM = 6, MN = 10, TN = 8, then Find LT.
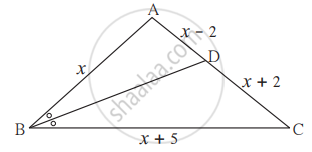
In ∆ABC, seg BD bisects ∠ABC. If AB = x, BC = x + 5, AD = x – 2, DC = x + 2, then find the value of x.
In ∆PQR seg PM is a median. Angle bisectors of ∠PMQ and ∠PMR intersect side PQ and side PR in points X and Y respectively. Prove that XY || QR.

Complete the proof by filling in the boxes.
In △PMQ, ray MX is bisector of ∠PMQ.
∴ `square/square = square/square` .......... (I) theorem of angle bisector.
In △PMR, ray MY is bisector of ∠PMQ.
∴ `square/square = square/square` .......... (II) theorem of angle bisector.
But `(MP)/(MQ) = (MP)/(MR)` .......... M is the midpoint QR, hence MQ = MR.
∴ `(PX)/(XQ) = (PY)/(YR)`
∴ XY || QR .......... converse of basic proportionality theorem.
In the given fig, bisectors of ∠B and ∠C of ∆ABC intersect each other in point X. Line AX intersects side BC in point Y. AB = 5, AC = 4, BC = 6 then find `"AX"/"XY"`.

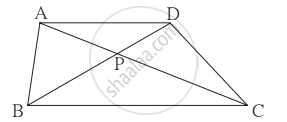
In ▢ABCD, seg AD || seg BC. Diagonal AC and diagonal BD intersect each other in point P. Then show that `"AP"/"PD" = "PC"/"BP"`.
In Δ ABC and Δ PQR,
∠ ABC ≅ ∠ PQR, seg BD and
seg QS are angle bisector.
`If (l(AD))/(l(PS)) = (l(DC))/(l(SR))`
Prove that : Δ ABC ∼ Δ PQR

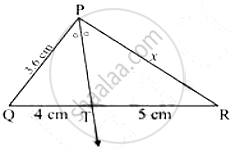
In the following figure, ray PT is the bisector of ∠QPR Find the value of x and perimeter of ∠QPR.
Draw the circumcircle of ΔPMT in which PM = 5.6 cm, ∠P = 60°, ∠M = 70°.
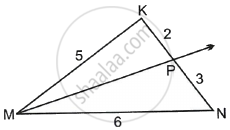
From the information given in the figure, determine whether MP is the bisector of ∠KMN.
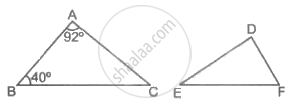
If ΔABC ∼ ΔDEF such that ∠A = 92° and ∠B = 40°, then ∠F = ?

In ΔABC, ray BD bisects ∠ABC, A – D – C, seg DE || side BC, A – E – B, then for showing `("AB")/("BC") = ("AE")/("EB")`, complete the following activity:
Proof :
In ΔABC, ray BD bisects ∠B.
∴ `square/("BC") = ("AD")/("DC")` ...(I) (`square`)
ΔABC, DE || BC
∴ `(square)/("EB") = ("AD")/("DC")` ...(II) (`square`)
∴ `("AB")/square = square/("EB")` ...[from (I) and (II)]
