Advertisements
Advertisements
प्रश्न
Find the equation of the circle whose centre is (2, 3) and which passes through (1, 4).
उत्तर
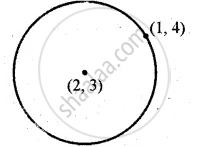
Centre (h, k) = (2, 3)
Radius = `sqrt((1 - 2)^2 + (4 - 3)^2)`
`= sqrt((-1)^2 + 1^2)`
`= sqrt2`
Equation of the circle with centre (h, k) and radius r is (x – h)2 + (y – k)2 = r2
⇒ (x – 2)2 + (y – 3)2 = `(sqrt2)^2`
⇒ x2 – 4x + 4 + y2 – 6y + 9 = 2
⇒ x2 + y2 – 4x – 6y + 11 = 0
APPEARS IN
संबंधित प्रश्न
Find the centre and radius of the circle.
(x + 2) (x – 5) + (y – 2) (y – 1) = 0
Find the Cartesian equation of the circle whose parametric equations are x = 3 cos θ, y = 3 sin θ, 0 ≤ θ ≤ 2π.
Find the length of the tangent from (1, 2) to the circle x2 + y2 – 2x + 4y + 9 = 0.
Find the value of P if the line 3x + 4y – P = 0 is a tangent to the circle x2 + y2 = 16.
Obtain the equation of the circle for which (3, 4) and (2, -7) are the ends of a diameter.
A circle of area 9π square units has two of its diameters along the lines x + y = 5 and x – y = 1. Find the equation of the circle
Choose the correct alternative:
The equation of the circle passing through (1, 5) and (4, 1) and touching y-axis `x^2 + y^2 - 5x - 6y + 9 + lambda(4x + 3y - 19)` = where `lambda` is equal to
Choose the correct alternative:
The length of the diameter of the circle which touches the x -axis at the point (1, 0) and passes through the point (2, 3)
Choose the correct alternative:
The radius of the circle 3x2 + by2 + 4bx – 6by + b2 = 0 is
Choose the correct alternative:
The radius of the circle passing through the points (6, 2) two of whose diameter are x + y = 6 and x + 2y = 4 is
