Advertisements
Advertisements
प्रश्न
Find the equation of the circle whose centre is (2, 3) and which passes through (1, 4).
उत्तर
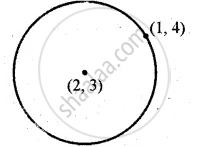
Centre (h, k) = (2, 3)
Radius = `sqrt((1 - 2)^2 + (4 - 3)^2)`
`= sqrt((-1)^2 + 1^2)`
`= sqrt2`
Equation of the circle with centre (h, k) and radius r is (x – h)2 + (y – k)2 = r2
⇒ (x – 2)2 + (y – 3)2 = `(sqrt2)^2`
⇒ x2 – 4x + 4 + y2 – 6y + 9 = 2
⇒ x2 + y2 – 4x – 6y + 11 = 0
APPEARS IN
संबंधित प्रश्न
Find the equation of the following circles having the centre (3, 5) and radius 5 units.
Find the values of a and b if the equation (a - 1)x2 + by2 + (b - 8)xy + 4x + 4y - 1 = 0 represents a circle.
If (4, 1) is one extremity of a diameter of the circle x2 + y2 - 2x + 6y - 15 = 0 find the other extremity.
The centre of the circle x2 + y2 – 2x + 2y – 9 = 0 is:
The equation of the circle with centre on the x axis and passing through the origin is:
If the perimeter of the circle is 8π units and centre is (2, 2) then the equation of the circle is:
If the circle touches the x-axis, y-axis, and the line x = 6 then the length of the diameter of the circle is:
A circle of area 9π square units has two of its diameters along the lines x + y = 5 and x – y = 1. Find the equation of the circle
Determine whether the points (– 2, 1), (0, 0) and (– 4, – 3) lie outside, on or inside the circle x2 + y2 – 5x + 2y – 5 = 0
Choose the correct alternative:
The circle passing through (1, – 2) and touching the axis of x at (3, 0) passing through the point
