Advertisements
Advertisements
प्रश्न
Find the image of the point (3, 8) with respect to the line x + 3y = 7 assuming the line to be a plane mirror.
उत्तर
Let the equation of line AB be x + 3y = 7 and the coordinates of point P are (3, 8).
y = `- 1/3 "x" + 7/3`
The image of point P will be Q if PQ ⊥ AB, PQ and AB intersect at the point M such that
PM = QM
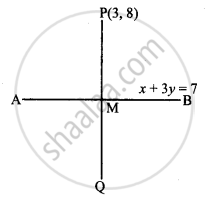
Slope of line AB = `-1/3`
And slope of PQ = 3
∴ Equation of line PQ,
y – 8 = 3(x – 3)
= 3x – 9
or 3x – y = 1 ….........(i)
Equation of AB x + 3y = 7 ….........(ii)
Multiplying equation (i) by 3 and adding it to equation (ii),
10x = 10 or x = 1
From equation (i) y = 3x – 1
= 3 – 1
= 2
∴ The coordinates of point M are (1, 2).
Let the coordinates of Q be (x1, y1)
Point M is the midpoint of line segment PQ
∴ While P(3, 8) is.
∴ `("x"_1 + 3)/2 = 1` or x1 = −1
`("y"_1 + 8)/2 = 2` or y1 = −4
∴ The image of P is (−1, – 4).
APPEARS IN
संबंधित प्रश्न
Find the equation of the line which satisfy the given condition:
Passing through the point (–4, 3) with slope `1/2`.
Find the equation of the line which satisfy the given condition:
Passing though (0, 0) with slope m.
Find the equation of the line which satisfy the given condition:
Passing though `(2, 2sqrt3)` and is inclined with the x-axis at an angle of 75°.
Find the equation of the line which satisfy the given condition:
Passing through the points (–1, 1) and (2, –4).
Find the equation of the line which is at a perpendicular distance of 5 units from the origin and the angle made by the perpendicular with the positive x-axis is 30°
Find the equation of the line passing through (–3, 5) and perpendicular to the line through the points (2, 5) and (–3, 6).
A line perpendicular to the line segment joining the points (1, 0) and (2, 3) divides it in the ratio 1:n. Find the equation of the line.
Find equation of the line passing through the point (2, 2) and cutting off intercepts on the axes whose sum is 9.
Find equation of the line through the point (0, 2) making an angle `(2pi)/3` with the positive x-axis. Also, find the equation of line parallel to it and crossing the y-axis at a distance of 2 units below the origin.
The perpendicular from the origin to a line meets it at the point (– 2, 9), find the equation of the line.
P (a, b) is the mid-point of a line segment between axes. Show that equation of the line is `x/a + y/b = 2`
Point R (h, k) divides a line segment between the axes in the ratio 1:2. Find equation of the line.
By using the concept of equation of a line, prove that the three points (3, 0), (–2, –2) and (8, 2) are collinear.
Find the area of the triangle formed by the lines y – x = 0, x + y = 0 and x – k = 0.
If the lines y = 3x + 1 and 2y = x + 3 are equally inclined to the line y = mx + 4, find the value of m.
Classify the following pair of line as coincident, parallel or intersecting:
2x + y − 1 = 0 and 3x + 2y + 5 = 0
Classify the following pair of line as coincident, parallel or intersecting:
3x + 2y − 4 = 0 and 6x + 4y − 8 = 0.
Prove that the lines 2x − 3y + 1 = 0, x + y = 3, 2x − 3y = 2 and x + y = 4 form a parallelogram.
Find the angle between the lines x = a and by + c = 0..
Prove that the area of the parallelogram formed by the lines a1x + b1y + c1 = 0, a1x + b1y+ d1 = 0, a2x + b2y + c2 = 0, a2x + b2y + d2 = 0 is \[\left| \frac{\left( d_1 - c_1 \right)\left( d_2 - c_2 \right)}{a_1 b_2 - a_2 b_1} \right|\] sq. units.
Deduce the condition for these lines to form a rhombus.
Show that the diagonals of the parallelogram whose sides are lx + my + n = 0, lx + my + n' = 0, mx + ly + n = 0 and mx + ly + n' = 0 include an angle π/2.
Show that the point (3, −5) lies between the parallel lines 2x + 3y − 7 = 0 and 2x + 3y + 12 = 0 and find the equation of lines through (3, −5) cutting the above lines at an angle of 45°.
Write an equation representing a pair of lines through the point (a, b) and parallel to the coordinate axes.
Let ABC be a triangle with A(–3, 1) and ∠ACB = θ, 0 < θ < `π/2`. If the equation of the median through B is 2x + y – 3 = 0 and the equation of angle bisector of C is 7x – 4y – 1 = 0, then tan θ is equal to ______.
