Advertisements
Advertisements
प्रश्न
Find the slope of the line passing through given points G(3, 7) and K(–2, –3).
उत्तर
Given points are G(3, 7) and K(–2, –3).
Let x1 = 3, x2 = – 2, y1 = 7, y2 = – 3
∴ Slope of line GK = `(y_2 - y_1)/(x_2 - x_1)`
⇒ Slope of line GK = `(-3 - 7)/(-2 - 3)`
= `(-10)/(-5)`
= 2
As a result, the slope of the line GK is 2.
APPEARS IN
संबंधित प्रश्न
Find x, if the slope of the line joining (x, 2) and (8, −11) is `−3/4`.
The side AB of a square ABCD is parallel to the x-axis. Find the slopes of all its sides. Also, find:
- the slope of the diagonal AC.
- the slope of the diagonal BD.
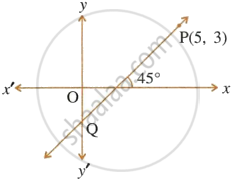
The line through P(5, 3) intersects y-axis at Q.
- Write the slope of the line.
- Write the equation of the line.
- Find the co-ordinates of Q.
Find the slope of the line which is perpendicular to `x - y/2 + 3 = 0`
Find the slope of the line which is perpendicular to `x/3 - 2y = 4`
If the lines y = 3x + 7 and 2y + px = 3 are perpendicular to each other, find the value of p.
Find the value of k for which the lines kx – 5y + 4 = 0 and 5x – 2y + 5 = 0 are perpendicular to each other.
Determine whether the following point is collinear.
A(–4, 4), \[K\left( - 2, \frac{5}{2} \right),\] N (4, –2)
If A(1, –1), B(0, 4), C(–5, 3) are vertices of a triangle then find the slope of each side.
Fill in the blank using correct alternative.
Distance of point (–3, 4) from the origin is ______.
Find the slope of a line, correct of two decimals, whose inclination is 60°
Find the slope of a line passing through the given pair of points (0,5) and (5,0)
Find the slope of a line parallel to the given line 5x-y = 10
The vertices of a triangle are A(10, 4), B(- 4, 9) and C(- 2, -1). Find the
Determine whether the following points are collinear. A(–1, –1), B(0, 1), C(1, 3)
Given: Points A(–1, –1), B(0, 1) and C(1, 3)
Slope of line AB = `(square - square)/(square - square) = square/square` = 2
Slope of line BC = `(square - square)/(square - square) = square/square` = 2
Slope of line AB = Slope of line BC and B is the common point.
∴ Points A, B and C are collinear.
