Advertisements
Advertisements
प्रश्न
Fill in the blank using correct alternative.
Distance of point (–3, 4) from the origin is ______.
विकल्प
7
1
5
−5
उत्तर
Distance of point (–3, 4) from the origin is 5.
Explanation:
Let the given point be P(–3, 4).
Distance of P(–3, 4) from the origin O(0, 0) is
`sqrt((0 - (-3))^2 + (0 - 4)^2`
= `sqrt(9 + 16)`
= `sqrt(25)`
= 5
APPEARS IN
संबंधित प्रश्न
The line through A(–2, 3) and B(4, b) is perpendicular to the line 2x – 4y = 5. Find the value of b.
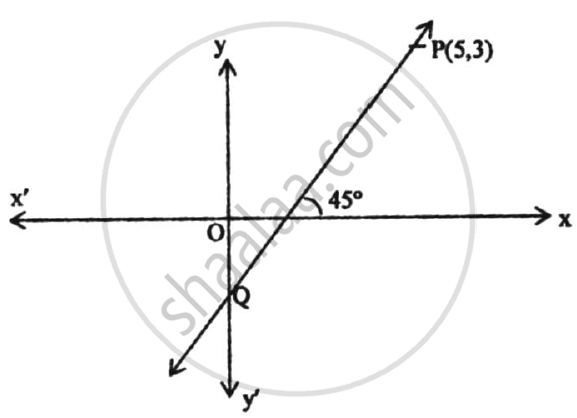
The line through P(5, 3) intersects y-axis at Q.
(1) Write the slope of the line.
(2) Write the equation of the line.
(3) Find the coordinates of Q.
A and B are two points on the x-axis and y-axis respectively. P (2, −3) is the midpoint of AB. Find the:
(1) coordinates of A and B
(2) slope of line AB.
(3) an equation of line AB.

Find the slope of the line parallel to AB if : A = (−2, 4) and B = (0, 6)
Find the slope of the line parallel to AB if : A = (0, −3) and B = (−2, 5)
Without using the distance formula, show that the points A(4, 5), B(1, 2), C(4, 3) and D(7, 6) are the vertices of a parallelogram.
Find the slope of the line which is parallel to `x/2 - y/3 -1 = 0 `
Find the slope of the line which is perpendicular to `x/3 - 2y = 4`
Lines 2x – by + 5 = 0 and ax + 3y = 2 are parallel to each other. Find the relation connecting a and b.
Lines mx + 3y + 7 = 0 and 5x – ny – 3 = 0 are perpendicular to each other. Find the relation connecting m and n.
Angle made by the line with the positive direction of X-axis is given. Find the slope of the line.
45°
If A(1, –1), B(0, 4), C(–5, 3) are vertices of a triangle then find the slope of each side.
Find k, if R(1, –1), S (–2, k) and slope of line RS is –2.
Find the slope of a line passing through the given pair of points (-5,-1) and (-9,-7)
Find the slope of a line parallel to the given line 3x-2y = 5
Find the slope of a line passing through the points (x, 9) and (12, 6) is `(-1)/3 = ("y"_2 - "y"_1)/("x"_2 - "x"_1)`
Find the slope and the y-intercept of the following line x - 2 = `(5 - 3"y")/2`
If A(6, 1), B(8, 2), C(9, 4) and D(7, 3) are the vertices of `square`ABCD, show that `square`ABCD is a parallelogram.
Solution:
Slope of line = `("y"_2 - "y"_1)/("x"_2 - "x"_1)`
∴ Slope of line AB = `(2 - 1)/(8 - 6) = square` .......(i)
∴ Slope of line BC = `(4 - 2)/(9 - 8) = square` .....(ii)
∴ Slope of line CD = `(3 - 4)/(7 - 9) = square` .....(iii)
∴ Slope of line DA = `(3 - 1)/(7 - 6) = square` .....(iv)
∴ Slope of line AB = `square` ......[From (i) and (iii)]
∴ line AB || line CD
∴ Slope of line BC = `square` ......[From (ii) and (iv)]
∴ line BC || line DA
Both the pairs of opposite sides of the quadrilateral are parallel.
∴ `square`ABCD is a parallelogram.
