Advertisements
Advertisements
प्रश्न
Find the value of x in the given figure.
उत्तर
∠B = 180° – 120° .....(Sum of the opposite angles of a quadrilateral are supplementary)
∠B = 60°
∠BCA = 90° ...(Angle in a semicircle)
∠BAC + ∠B + ∠BCA = 180°
x + 60° + 90° = 180°
x + 150° = 180°
x = 180° – 150°
= 30°
The value of x = 30°
APPEARS IN
संबंधित प्रश्न
Prove that the “the opposite angles of the cyclic quadrilateral are supplementary”.
In the given figure, ▢PQRS is cyclic. side PQ ≅ side RQ. ∠PSR = 110°, Find -
(1) measure of ∠PQR
(2) m(arc PQR)
(3) m(arc QR)
(4) measure of ∠PRQ
`square`MRPN is cyclic, ∠R = (5x – 13)°, ∠N = (4x + 4)°. Find measures of ∠R and ∠N.
Prove that, any rectangle is a cyclic quadrilateral
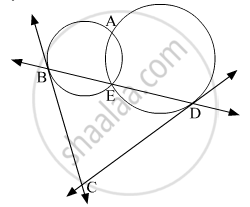
In the given figure, two circles intersect each other at points A and E. Their common secant through E intersects the circles at points B and D. The tangents of the circles at points B and D intersect each other at point C. Prove that ▢ABCD is cyclic.
In the given figure, seg AD ⊥ side BC, seg BE ⊥ side AC, seg CF ⊥ side AB. Ponit O is the orthocentre. Prove that , point O is the incentre of ∆DEF.

A school wants to conduct tree plantation programme. For this a teacher allotted a circle of radius 6 m ground to nineth standard students for planting sapplings. Four students plant trees at the points A, B, C and D as shown in figure. Here AB = 8 m, CD = 10 m and AB ⊥ CD. If another student places a flower pot at the point P, the intersection of AB and CD, then find the distance from the centre to P.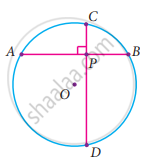
MRPN is cyclic, ∠R = (5x – 13)°, ∠N = (4x + 4)°. Find measures of ∠R and ∠N, by completing the following activity.
Solution:
MRPN is cyclic
The opposite angles of a cyclic square are `square`
∠R + ∠N = `square`
∴ (5x – 13)° + (4x + 4)° = `square`
∴ 9x = 189°
∴ x = `square`
∴ ∠R = (5x – 13)° = `square`
∴ ∠N = (4x + 4)° = `square`
Prove the following theorems:
Opposite angles of a cyclic quadrilateral are supplementary.
In the figure, PQRS is cyclic, side PQ ≅ side RQ, ∠PSR = 110°. Find

(i) measure of ∠PQR
(ii) m(arc PQR)
(iii) m(arc QR)
