Advertisements
Advertisements
प्रश्न
If AB is a chord of a circle, P and Q are the two points on the circle different from A and B, then
विकल्प
∠APB = ∠AQB
∠APB + ∠AQB = 180° or ∠APB = ∠AQB
∠APB + ∠AQB = 90°
∠APB + ∠AQB = 180°
उत्तर
∠APB + ∠AQB = 180° or ∠APB = ∠AQB
We are given AB is a chord of the circle; P and Q are two points on the circle different from A and B.
We have following figure.
Case 1: Consider P and Q are on the same side of AB
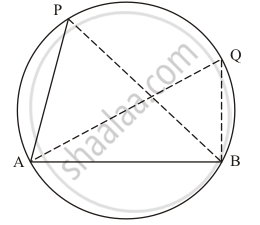
We know that angle in the same segment are equal.
Hence, ∠APB = ∠AQB
Case 2: Now consider P and Q are on the opposite sides of AB
In this case we have the following figure:
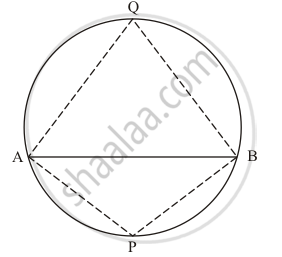
Since quadrilateral APBQ is a cyclic quadrilateral.
Therefore,
∠APB + ∠AQB = 180° (Sum of the pair of opposite angles of cyclic quadrilateral is 180°.)
Therefore, ∠APB = ∠AQB or ∠APB + ∠AQB = 180°
APPEARS IN
संबंधित प्रश्न
If a line intersects two concentric circles (circles with the same centre) with centre O at A, B, C, and D, prove that AB = CD (see given figure).

A circular park of radius 20m is situated in a colony. Three boys Ankur, Syed and David are sitting at equal distance on its boundary each having a toy telephone in his hands to talk each other. Find the length of the string of each phone.
true or false
If a circle is divided into three equal arcs each is a major arc.
Find the length of a chord which is at a distance of 5 cm from the centre of a circle ofradius 10 cm.
Angle formed in minor segment of a circle is
In a circle with centre O, AB and CD are two diameters perpendicular to each other. The length of chord AC is
Two equal circles of radius r intersect such that each passes through the centre of the other. The length of the common chord of the circle is
Two chords AB and CD of a circle are each at distances 4 cm from the centre. Then AB = CD.
Two chords AB and AC of a circle with centre O are on the opposite sides of OA. Then ∠OAB = ∠OAC .
Two congruent circles with centres O and O′ intersect at two points A and B. Then ∠AOB = ∠AO′B.
