Advertisements
Advertisements
प्रश्न
If AB is a chord of a circle, P and Q are the two points on the circle different from A and B, then
पर्याय
∠APB = ∠AQB
∠APB + ∠AQB = 180° or ∠APB = ∠AQB
∠APB + ∠AQB = 90°
∠APB + ∠AQB = 180°
उत्तर
∠APB + ∠AQB = 180° or ∠APB = ∠AQB
We are given AB is a chord of the circle; P and Q are two points on the circle different from A and B.
We have following figure.
Case 1: Consider P and Q are on the same side of AB
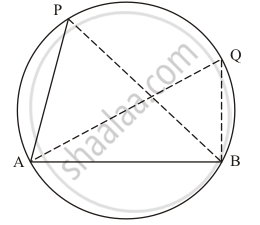
We know that angle in the same segment are equal.
Hence, ∠APB = ∠AQB
Case 2: Now consider P and Q are on the opposite sides of AB
In this case we have the following figure:
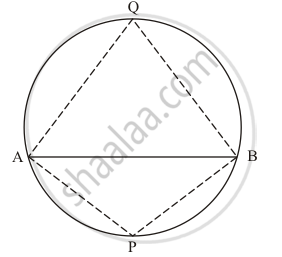
Since quadrilateral APBQ is a cyclic quadrilateral.
Therefore,
∠APB + ∠AQB = 180° (Sum of the pair of opposite angles of cyclic quadrilateral is 180°.)
Therefore, ∠APB = ∠AQB or ∠APB + ∠AQB = 180°
APPEARS IN
संबंधित प्रश्न
Two circles of radii 5 cm and 3 cm intersect at two points and the distance between their centres is 4 cm. Find the length of the common chord.
If two equal chords of a circle intersect within the circle, prove that the segments of one chord are equal to corresponding segments of the other chord.
If two equal chords of a circle intersect within the circle, prove that the line joining the point of intersection to the centre makes equal angles with the chords.
If a line intersects two concentric circles (circles with the same centre) with centre O at A, B, C, and D, prove that AB = CD (see given figure).

Find the length of a chord which is at a distance of 4 cm from the centre of the circle of radius 6 cm.
An equilateral triangle of side 9cm is inscribed in a circle. Find the radius of the circle.
If two diameters of a circle intersect each other at right angles, then quadrilateral formed by joining their end points is a
In a circle with centre O, AB and CD are two diameters perpendicular to each other. The length of chord AC is
Two congruent circles with centres O and O′ intersect at two points A and B. Then ∠AOB = ∠AO′B.
If two equal chords of a circle intersect, prove that the parts of one chord are separately equal to the parts of the other chord.
