Advertisements
Advertisements
प्रश्न
In a circle with centre O, AB and CD are two diameters perpendicular to each other. The length of chord AC is
विकल्प
2AB
- \[\sqrt{2}\]
- \[\frac{1}{2}AB\]
- \[\frac{1}{\sqrt{2}}AB\]
उत्तर
We are given a circle with centre at O and two perpendicular diameters AB and CD.
We need to find the length of AC.
We have the following corresponding figure:
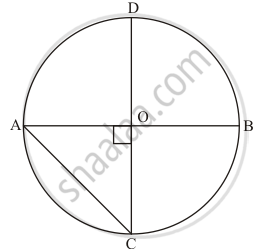
Since, AB = CD (Diameter of the same circle)
Also, ∠AOC = 90°
And, AO = `(AB)/2`
Here, AO = OC (radius)
In ΔAOC
`AC^2 = AO^2 + OC^2 = AO^2 + AO^2`
`= ((AB)/2)^2 + ((AB)/2)^2`
`AC^2 = (AB^2)/2`
`AC = (AB)/(sqrt(2))`
APPEARS IN
संबंधित प्रश्न
If two equal chords of a circle intersect within the circle, prove that the line joining the point of intersection to the centre makes equal angles with the chords.
A circular park of radius 20m is situated in a colony. Three boys Ankur, Syed and David are sitting at equal distance on its boundary each having a toy telephone in his hands to talk each other. Find the length of the string of each phone.
Find the length of a chord which is at a distance of 5 cm from the centre of a circle ofradius 10 cm.
Find the length of a chord which is at a distance of 4 cm from the centre of the circle of radius 6 cm.
An equilateral triangle of side 9cm is inscribed in a circle. Find the radius of the circle.
If two diameters of a circle intersect each other at right angles, then quadrilateral formed by joining their end points is a
Angle formed in minor segment of a circle is
In a circle of radius 17 cm, two parallel chords are drawn on opposite side of a diameter. The distance between the chords is 23 cm. If the length of one chord is 16 cm, then the length of the other is
Two chords AB and AC of a circle with centre O are on the opposite sides of OA. Then ∠OAB = ∠OAC .
AB and AC are two chords of a circle of radius r such that AB = 2AC. If p and q are the distances of AB and AC from the centre, prove that 4q2 = p2 + 3r2.
